Has anyone who wrote this actually passed calculus?

The law implies that students have the agency to enroll in pretransfer class.

The APY is NOT also called the APR. See https://www.investopedia.com/personal-finance/apr-apy-bank-hopes-cant-tell-difference/

This really be a stated as a *repeating" pattern. For example, 1.01001000100001000001... has a pattern, but it is not rational.

I don't know why this section put such emphasis on Standard From of a linear equation. It could also use more example of finding an equation of a line given slope and a point that's not the y-intercept.

This cannot be 5 when you add 1 and 2. You get 3. For a digit to be 5, you must have at least base 6. The digit 3 is allowed in base 6 or higher, so the 5 can only be 3, assuming the 1 and 2 are not typos.

Instead of writing 14 above the 4, don't we usually just write a 1 to the left of 4 to make 14?

This paragraph should be revised:

The above method is sometimes called the Complementary Method. There is yet a more specific approach called the Complementary Algorithm. It relies on the idea of complements, where two values are complements when their sum is a specified value. In Base Ten, pairs of complements were 1 & 9, 2 & 8, 3 & 7, 4 & 6 and 5 & 5, because each pair sum to the value 10. For the complementary method, you find a very specific complement of the subtrahend and add it to both the minuend and subtrahend before subtracting. In the complementary algorithm, the complements sum to a 1 followed only by zeros such that the number of zeros d is the same number of digits in the minuend, i.e., the complements sum to \(10^d\).

Distance between two values is the magnitude of the value to add to go from one number to another. However, the example really doesn't match the description in Figure 3.2.1.

Inverse operation: To find out what was added to get 10, start from 10 and find out what needs to be subtracted to get back to 7.

Explain why this works. For each division statement, interpret the quotient and reminder with blocks.

Illustrate with virtual base blocks.

Should list the combinations that didn't work.

The link at the end is supposed to point to https://learning.ccsso.org/common-core-state-standards-initiative, and it should be http://www.corestandards.org.

Generalize this to different number of coins.

Good extension problem after counting triangles in this star-in-pentagon diagram.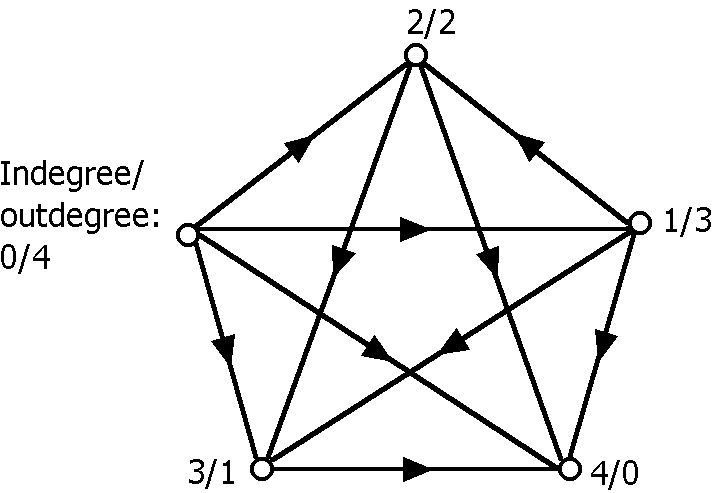

Also extend to n by n board.

A good use of technology to check adding consecutive numbers. Mathematical Practice #5

Also a good extension after the equilateral triangle problem.

This should be separate page. Not related to "Student Thinking."

An example is when we think geometrically about algebra. For instance * Geometric interpretation of reciprocal of squares * How naive fraction addition results in a fraction that is between the original two fraction

What are the assumptions in this question? 1. We are looking to use as few cats as possible to kill 100 rats as close to 50 min as possible. 2. Do all the cats kill rats independently, or do all the cats work together to kill one rat at a time?

Another indication that the authors don't have experience teaching math to a wide range of students. Have they not observe how prevalent it is that students tune out explanations and go straight to formulas and mimicking?

Have the authors taught math in classroom to observe that students often don't pay attention during direct instruction? What if small group is where learning occurs and masking the ineffectiveness of DI when the two are used together, which is often? Independent problems and projects are often not done independently--tutors, asking friends, copying.

Use the follow-up tag to delve further.

More effective and efficient under what metric? Are those metric aligned with the value of mathematical practices besides regurgitating the content? Will need to follow up with the citation.

This is totally against the spirit of DOING mathematics. How are students to learn what it means to engage in mathematical practice without engaging in mathematical practice?

How does the proportion change when focusing on CCC STEM students?

This one year time frame seems limiting. With still a large proportion of non-success in direct placement, resulting in students who have experienced no math success in a whole year, do these non-success students achieve at the same rate as those taking transfer-level for the first time or are they much worse, allowing the other group to catch up in the second year?

What does this mean, "taking into account"? How would the "6.7 times" later in the sentence change if "not taking into account"?

What percent of students did not complete Algebra 1 in the study? It seems odd that we switch the frame from students who did not completing Algebra 2 to those who completed Algebra 1. Why not continue with the same language?

For the 62% that start in transfer-level but had not experienced success for two consecutive semesters, what happens to them? What's their success rate on their next attempt?

What's the success rate starting and passing Intermediate Algebra in one year? What is their success rate on their first attempt at transfer-level BSTEM after passing Int Alg and how does that compare with those who failed transfer-level twice in a row?

But what is the success rate of Intermediate Algebra by the end of one year?

What is the throughput after two years? One-year throughput rate of less than 40% still leaves more than 60% behind. How do those 60% compare with those who took a year to complete Intermediate Algebra in completing transfer-level course in their third semester? The prior group had two failures, while the latter group had a failure and a success.

That "level of efficacy" refers to the 55% in the previous paragraph. If natural immunity has this efficacy, then it should be considered pretty good, no?

Let:<br> Rv = risk of infection for vaccinated folks not previously infected<br> Ru = risk of infection for unvaccinated folks not previously infected<br> Rvc = risk of folks who are vaccinated after prior infection<br> Ruc = risk of folks who are not vaccinated after prior infection

The highlight result means that Ruc = 5Rv

Assuming a vaccine has 95% efficacy, that means the risk of infection among unvaccinated is 20 times higher than those who are vaccinated. So Ru = 20Rv = 4(5Rv) = 4 Ruc --> 0.25 = Ruc/Ru. This means that natural immunity confers about 75% efficacy against infection.

When the vaccines were first undergoing efficacy studies, scientists were expecting 55%, so natural immunity at 75% isn't considered an ineffective number.

But wouldn't a more fair comparison be comparing with those who got vaccinated but not had COVID? The first level question about natural immunity is whether or not it is comparable to vaccination.

Let Rv = risk of infection for vaccinated folks not previously infected,<br> Ru = risk of infection for unvaccinated folks not previously infected,<br> Rvc = risk of folks who are vaccinated after prior infection<br> Ruc = risk of folks who are not vaccinated after prior infection.

Assuming a vaccine has 95% efficacy, that means the risk of infection among unvaccinated is 20 times higher than those who are vaccinated. So Ru = 20Rv The result of the present study gives 2Rvc < Ruc < 3Rvc

It seems reasonable to assume that Rv >= Rvc, since the prior COVID infection gave the immune system a jump start.

Now let's estimate Ruc/Ru:<br> $$Ru = 20Rv\ge 20Rvc = 20/3(3Rvc) > (20/3)Ruc$$<br> So \(Ruc/Ru < 3/20\). This gives natural immunity at least 85% efficacy against reinfection.

If Rv <= Rvc for some reason, Ru = 20Rv <= 20Rvc = 10(2Rvc) < 10Ruc. Ruc/Ru > 1/10. This gives natural immunity at best 90% efficacy against reinfection.

Thus, if Rv ~ Ruc, then natural immunity has around 85-90% efficacy.

Important!