Needs a third sub diagram that shows the reversed current.

Would be a restoring force, leading to oscillation, if current continues in the initial direction.

...using tons of trig and calculus!

Important diagram

We will try this demonstration in Friday's session 23.

Cf., The Hunt for Red October.

https://www.imdb.com/title/tt0099810/

Will review this in Session 23.

We viewed this force effect in Session 21, with the big horseshoe magnet and the thick copper strip between the poles of the magnet.

We will work out an example from this set up in our Session 21.

Bypass

For Exam 2, we will bypass the aurora and other examples past this point.

Like in CSI

Cosmic rays are protons and other particles blown out by supernova detonations and big galactic black holes.

I severely hate this version of the right hand rule. However, other semingly normal people might like it, so I will include it in the textbook without mass crossouts.

"relatively simple" ← nice, but do not forget the appearance/disappearance of the magnetic field depending on the motion of the observer.

This is a good set of questions to ask of the magnetic field of Earth. Theoretical image of field lines:

Actual field lines:

Very important diagram.

As reviewed in Session 20.

Always true, unlike electric field lines. There are electric monopoles, e.g., proton and electron, with porcupine and anti-porcupine field lines. There are looped \(\vec{E}\) field lines starting with a dipole array.

Ditto with \(\vec{E}\) field lines

Flux again.

Good -- similar to our idea about electric field lines.

NOT

Will mention in Session 20 or 21.

crude indeed. As far as we can tell, electrons have no structure, so saying that it spins on an axis like a planet is very crude. We make do, saying that the electron has intrinsic spin angular momentum, \(\plusmn \frac{\hbar}{2}\)

Moving charge is the source of the magnetic field.

Any large structure built of steel, like a building of steel girders or a warship, can be magnetized by pounding the steel: pile drivers, riveters, etc.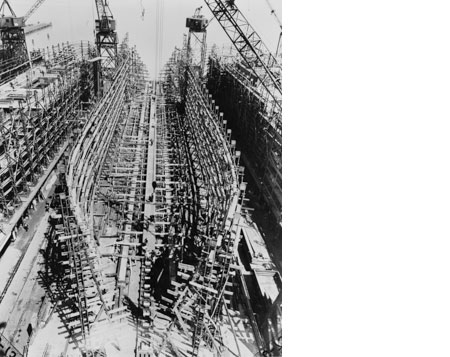 Liberty ship, keel plates

This topic should follow "Electromagnets" because we understood electromagnets a century earlier than we savvied ferromagnetism (iron atoms as tiny electromagnets).

Check for paired white-and-black spots on the Sun's magnetogram. The Sun is pretty quiet now, however.

So the magnetic pole up in arctic Canada is actually an S!!

Might be no such thing as a magnetic monopole. Magnets seem only to come as dipoles.

We can thank Michael Faraday for these developments.

WHat an understatement! Transistors on various electronics chips are on the order of a few dozen nanometers!

Check the Session 18 extension in YouTube.

The circuit's "time constant" \(\tau = RC\) is related to the half-life of the circuit. Both terms are used by scientists in exponentially growing or decaying systems.

Will be helpful when working on HW 2.

We will work out a few like this on Friday, 10/4.

idealization

So after an amount of time \(\Delta t\), all of the energy of a battery will dissipate at the circuit element, e.g., the light bulb, where stored Joules from the battery are dissipated as light and heat. At that point, the bulb goes cold.

Conservation of current.

better

NO! NO! NO! Voltage is measured in \(\frac{Joule}{Coulomb}\) but power is different, \(\frac{Joule}{second}\)!!

Nice definition

We will have discussions about these diagrams on Friday, 10/4.

This term traces way back to the 1800s, before we had figured out everything and unified the electromagnetic theory. So calling it a force is anachronistic, but we still use it for the sake of custom. The common symbol is an upper case script E, \(\mathcal{E}\)

This is what scientists and engineers call \(I^2R\) heating, a.k.a., Joule heating. Cf., Ch. 21.3

Mentioned in Lecture 14, Fall 2019

This was as far as the reading went to prepare for Exam 1. The sections after this will be on Exam 2!

We will work out this exercise in Session 13, if possible

a pipe filled with gravel

more current that gets through to the device, less resistance.

Dissipation of energy into the metallic lattice of the conductors, not to the hair dryer or other device hooked up to the circuit.

For instance, in the solar corona, positive ions, protons and electrons are flying around in "current sheets" blazing through the corona, and we have a hard time figuring out the Ohmic resistance for such complicated currents. YouTube

Will work this out in Session 13

Good problem to try out.

also out of place

This problem is slightly out of place... might be better in a later section of Ch. 20

information....

Big number, \(8.342\times 10^{28}\), but a few feet of 12 gauge wire is not nearly one cubic meter.

A specification of manufacture.

We will work through a calculation like this in Session 13, 9/25/19.

a lot like water, which is pretty much an incompressible fluid

less than 1 millimeter per second!

$$c=3\times 10^8 \frac{m}{s}$$

We can do all of our calculations etc. with conventional current \(I\left(t\right)\), but when actually trying to understand what is physically moving so that you can capture it or track it, you have to think about electrons, if you are in a regular circuit. Electrons require "reverse psychology."

and everyone else until about 1899

E.g., Kenmore 81422 washer/dryer, minimum circuit rating: 20 Amps.

Cf., for example, the BP-ATM-15-RP fuse for Ford F-150,

rated with amperage 15 Amps.

refer to Fig. 1

"...rate at which charge flows" past a given point in a conductor, $$\frac{\Delta q}{\Delta t}$$

although the photo is a water current! 🤦🏻‍♂️

The authors should title this section, "19.7 Energy Stored in Defibrillators" ; )

basic

A basic question

This and the following few sentences are describing a calculus equation using words instead of formulas.

This formula, \(E=\frac{1}{2}\frac{1}{C}Q^2\) is actually related to oscillating currents in a circuit, as we shall see in Ch. 23.

Energy delivered from a defibrillator's cap. E.g., "At 200 J, the ZOLL RBW delivers more average current to high-impedance patients than any other biphasic waveform."

Zoll makes all kinds of defibrillators, rated in Joules.

aka, sinus rhythm,

Hmmmmmm...This would make a lovely iClicker item.

Uh oh

A brain burner.... work this out before Monday lecture if you can!!!!!!

First two capacitors are parallels: \(10\mu F\) and \(2.5\mu F\), so they simply add up the preliminary combo capacitance: \(C_{12}=12.5\mu F\). Easy.

However, there is one cap in series with those first two, so now you employ the reciprocals formula for the total equivalent capacitance \(C_{eq}\), viz.

$$\frac{1}{C_{eq}}=\frac{1}{C_{12}}+\frac{1}{0.30\mu F}$$

So the common denominator is semi-nasty, \(cd=\left(12.5\right) \left(0.3\right) \longrightarrow 3.75 \)

The rest is fractions. Don't forget to flip your \(\frac{1}{C_{eq}}\) fraction to get \(C_{eq}\) itself.

We will get into this kind of brain burner on Monday, plus in a lab this coming week.

Easier

The top of three capacitors are equivalent to one big plate, and same for the bottoms.

must use common denominators!

For \(n\) capacitors in series, $$\frac{1}{C_{eq}}=\frac{1}{C_1}+\frac{1}{C_2}+\cdots+\frac{1}{C_n}$$

...or in Dr. Brueckner's parlance...

$$\Delta V=\frac{Q}{C_{eq}}=\frac{Q}{C_1}+\frac{Q}{C_2}+\frac{Q}{C_3}$$

• First capacitor, \(\Delta V_1=\frac{Q}{C_1}\)
• Second capacitor, \(\Delta V_2=\frac{Q}{C_2}\)
• Third capacitor, \(\Delta V_3=\frac{Q}{C_3}\)

Note that the assumed charge load at the top of the first capacitor and at the bottom of the third capacitor are assumed to be the same size, \(\pm Q\). This is an application of the conservation of charge: the number of electrons stolen from the top plate is the same as the load of surplus electrons pushed into the bottom plate.

Total voltage drop = \(V\) of the battery! But this time it is split up between three separate capacitors

A single equivalent capacitance, \(C_{eq}\)

Pretty nifty simulation. I tracked the electric field line densities for various dielectrics offered. Have a look at this simulation

First mentioned in Session 8.

Nice

Household size units for capacitors. Go into Radio Shack and check them out.

This property, permittivity, is related to a substance's ability to be polarized. You can look up the specs for permittivity for many substances, e.g., for muscle fiber. However, the vacuum also takes a value, denoted \(\epsilon_0\) and used here for parallel plates, even though the vacuum has no substance.

I detest this description, but it sort of makes some sense.

...from the battery

Fried dielectric time!

Many studies of dielectric properties, biological and otherwise.

Breakdown:

1. Sparks if vacuum or air filled capacitor;
2. fried dielectric if filled with a dielectric material like paper or paraffin.

For a singly ionized sodium atom, the time to cross this membrane is about \(\Delta t = 0.02\: ns\)... pretty quick!

As mentioned in lecture. For those of you studying biology, this is an important application

This is why the authors show the rolled up capacitor in Fig. 1

flux concept

Can be approximated as parallel plates, with an insulating layer in between.

They also store energy in the electric field. This is the first time we have had something other than a Sir Isaac Newton \(F=ma\) style object with mass that can carry energy.

A great paragraph about what a capacitor is and how it works

The ancient Greeks could not measure with this precision, nor could the great Tycho Brahe. Kepler and Galileo, Newton and Halley: nope. But by the 1800s, yup, it started becoming possible, with better telescopes and measuring devices.

This is the end of the material in Ch. 19 needed for Exam 1.

True, but astronomers still use both pc and LY!

Parallax angle P, in the diagram

Actually, a successful parallax measurement would've satisfied Plato, Aristotle et al., in their day, that Earth orbited the Sun. Unfortunately, they did not have good enough technology. But neither did Kepler and Galileo. Bessel bagged 61 Cygni 200+ years after Galileo.

This is essentially what astronomers do when they make pallalax measurements. But for stars, it is a much larger triangle.

I like this question. It is not a calculation, but it would be very nice to ask in class.........hmmmmm

A lot of sketching.... for after Exam 1!!

Very interesting

We talked about this a lot in Session 9.

A nice sketching exercise, equipotential lines of a dipole field. We might go through this in our first session after Exam 1.

Huge amount of difficult calculus behind this paragraph, enough to choke Sir Isaac Newton's horse.

Correct. In a conducting material, the surplus charges will redistribute themselves into dynamic equilibrium, equal distances from each other.

from PHY2053!!

True in general for small patches of spacetime, as I mentioned at the end of Session 9.

Discussed in Session 9

Visual way of thinking about the electromagnetic field, and very useful in quantum mechanics.

Does this diagram remind you of a hydrogen atom/Quantum_Mechanics/09._The_Hydrogen_Atom/Bohr's_Hydrogen_Atom)?

As I mentioned in an earlier annotation.

Bypass this example

Bypass this example

Just as we have the freedom to choose the elevation at which \(GPE=0.0\: J\) in a free fall problem near the surface of Earth: gym floor for a basketball problem, foot of a cliff for a Ferrari driving off a cliff problem, or the top of the same cliff for the Ferrari problem.

NASA will take \(GPE=0.0\: J \text{ as } r \longrightarrow \infty \) since they are ranging way past the surface of Earth and out into space.

One of the most annoying phrases in a physics textbook, "it can be shown that..." It is an author's evasive maneuver to avoid pages and pages of nasty calculus equations. Fortunately, that is okay in PHY2054!

Like the top of a Van de Graaff generator

Cf., Session 9 in YouTube

Yeah, the old timey method for generating a tv picuture, in the old tv tubes you used to see before flat screen technology took over. and The gun is in back, targeted at the front tv screen.

I.e., ripping outer electrons from \(O_2\), \(N_2\) and \(H_2 O\) molecules, making them into conduction electrons. BANG!

smooth versus sharp surfaces... Notice that insulators on telephone poles are nicely rounded ceramic or glass: and here is a closeup:

To encode directionality, should be $$\vec{E}=-\frac{V}{d}$$\ or better yet, $$\vec{E}=-\frac{\Delta V}{\Delta s}$$

...but the authors are only talking about magnitudes here.

Include this with my comments on units in Session 9.

...for this parallel plates example only. In general, one could have any kind of parabolic path between the plates, just as between elevations \(y=0.00\: m\) and \(y=40.0\: m\) from the top of the UCF library to the sidewalk, you could have any number of differing parabolic trajectories for a water balloon, depending on how hard you throw it and in what direction.

This is another nice exercise.

Good. I like this basic problem

Important example

What does it mean for a battery to "move 5000 Coulombs"? A battery is rated in Amp hours, e.g.,

An amp hour is a unit of charge: $$1.00\:A \times 1.00\:hour$$ $$1.00 \text{Coulomb}/\text{sec} \times 3600\:sec$$ $$3600 Coulombs$$

discussed in Session 8

Similar to the customary approach to GPE in a free fall system. You can define any elevation you like to be the zero point of the potential energy function, $U$... $$U\left(y\right)=-mg\Delta y$$

A scalar-valued function of position, though a ton of trig is usually encoded in the function

Emphasis on "as if" !!! ...because of the ambiguity in forces and potential energies that rises from having positive and negative charges

Further abstraction. \( \Delta V=-E \Delta x \) in a uniform field \(E\), e.g., between parallel charged plates. Does that formula remind you of the work formula?

Each has the same voltage -- determined by the cource charge array, not the apparatus (motorcycle vs. car)

ionic pumps

Reviewed in Session 8

BANG! Another exercise I like.

Yes, I like this exercise, too.

I like this exercise

Another flux law

Useful for visually interpreting the curvature of field lines.

also a flux law

Although Michael Faraday at first DID think that field lines were physical. He called them lines of force.

A flux law. If we were using calculus, there would be a huge calculus equation for this law, but the verbal form here is perfectly righteous and useful.

OH MY GOODNESS!

A brain burner!!!

Not sure what this is asking. BYPASS

We worked on similar calculations in class.

Fairly easy to calculate, if you know the value of Coulomb's constant, \(k\)

I like this one, a good basic workout.

Theoretically, an inverse r² force has infinite range. So: gravitation and electromagnetism.

Caveat: electromagnetic interactions can be screened, like losing your cell phone signal inside certain buildings on campus.

We reviewed this in Session 5, 09/06

Nice exercise, relating the metric unit of charge, the Coulomb, to the fundamental charge,

e=1.602 x 10^-19 Coulomb

Hint: if its net charge is negative, then it has EXTRA electrons.

A brain burner!

I like this question.

I like this exercise. It is a cool demonstration you can do at home!

1. Turn on the water to the lowest setting at which water streams out without forming droplets;
2. charge up a plastic comb by scuffling it on your shirt;
3. ease the comb in close to the vertical stream of water...

...How do you explain the fact that the stream dips slightly toward the comb (as in the photo) and never away from the comb?

The electrons at the left hand side of the grey conducting block flee the copper colored rod, which is net negative. Those fugitive electrons head over to the right hand side of the conducting block. POLARIZED!

This is basically what happened with the soda pop can demonstration.

Earth is negatory

polarize, then separate

positive charges "move" to the sharpest topography of the device, just as they move to a students hair when touching the Van de Graaff generator.

e.g., some of the valence electrons

...as opposed to pure water, no dissolved minerals, which is actually a fair insulator.

Demonstrations in Session 7, Sept. 11

very "sharp" compared to other parts of the body

We mentioned this in Session 7

i.e., air

Plate width \(W\gg d\) the distance between plates. Normal batteries and capacitors rely on this limit.

More statements based on flux.

Yes, important when applied to wires in a circuit.

This is why the aluminum soda pop can rolled in Session 7 demonstration

Here is a better model than Fig. 2 <script async src="//embedr.flickr.com/assets/client-code.js" charset="utf-8"></script>

Membranes and batteries and capacitors have similar structure.

hydrogen bonds

Mentioned in terms of complex organization, Session 7.

This statement and surrounding statements mentioned in HW 03.

Spherical symmetry, very handy, though lots of trig is possible. All complicated fields, like the electric field of a charged metal plate in a battery, is built up of zillions of point charge fields (using calculus and trig for days).

Very important. This cannot be done with forces. Cf., Session 7

Again, the conundrum of hands touching the generator but the strands of hair displaying the electrical effect.

The author is being tricky here. QUarks are subatomic particles with partial charges, The up quark (u) has charge $$+\frac{2}{3}e$$ and the down quark (2) has fractional charge \(-\frac{1}{3}e\)

1. neutron = dud
2. proton = uud

However, quarks are hard to pull out of a proton or neutron and have never been observed in isolation. *

$$6.25\times 10^{18}\text{ electrons}\longrightarrow -1.00\:Coulomb$$

E.g., muons that form from cosmic rays.

Cf., cosmic ray interactions with the Earth's atmosphere.

i.e., neutral atoms, not ions

The electromagnetic interaction is trickier than the gravitational interaction, because

• there are two kinds of charge, and
• there is repulsion as well as attraction.

For this reason, deciding the net electrical force on an electron or a proton, or an array of electrons or an array of protons, can be inticate, accounting-wise, because you have to account for

1. the geometry of the array,
2. the sign of the charges, and
3. the repulsion or attraction of the charges on each other

The Greek word for amber is ήλεκτρο, from which we get the word electric, electron etc.

Actually, we now have a great unifying theory for electromagnetic and weak nuclear interactions. It is called "electroweak theory." We won't be studying it, but it is out there. Here is something from Georgia State Hyperphysics You can take a peek at it, read around if it strikes your fancy.

If the sliding motion is the seat of this little kid's pant, then why do excess positive charges end up along each strand of hair?

We will understand this conundrum directly.