Basically, if we can obtain a spectrum of a galaxy, we can immediately tell how far away it is.
Yes, definitely handy, because if we make a big telescope we can see and catch spectra of really distant, faint galaxies!
E.g., from Kirshner, PNAS, 2004,
spectra of galaxies contained a wealth of information about the composition of the galaxy and the velocities of these great star systems.
How it was discovered
Hubble’s Law
Bypass
Models for an Expanding Universe
Bypass My balloon analogy in Mini-Lecture C is better.
the spectral lines of most galaxies showed an astounding redshift.
BINGO! An unexpected result.
a redshift is seen when the source of the waves is moving away from us
d is the distance
distance as derived from, e.g., Cepheid variables and Type Ia supernova measurements.
just as Lemaître had suggested.
Lemaître was correct!!!! It forced Einstein to say that his own model of all galaxies was the biggest scientific mistake of his life.
the universe is expanding.
Variation of Hubble’s Constant
Bypass
the evidence for a supermassive black hole at the center of the Galaxy is convincing indeed.
less than the diameter of Mercury’s orbit.
It works out to about \(R=46 \,LS\), which would be well inside Mercury's orbit, \(0.39\, AU=193\, LS\)
There are also thousands of stars within a parsec of Sagittarius A*.
There are only a few stars within a parsec of our solar system.
First, an asteroid might have ventured too close to the black hole and been heated to a very high temperature
Nifty. This is like seeing a mega-Chelyabinsk event crush into the black hole... seeing it from halfway across the galaxy.
One of the stars has been observed for its full orbit of 15.6 years.
S2
As wavelength decreases, they shift toward the blue end of the spectrum
The wavelengths of the absorption lines can be measured accurately, however, and their Doppler shift is relatively simple to detect.
absorption OR emission lines can display redshift and blueshift
difference in wavelength
I.e., \(\frac{\Delta \lambda}{\lambda}\)
That’s really an impressive amount of information for stars that are light-years away.
Color Shifts
This is the main concept to focus on, for Doppler shifting. Redshift and blueshift are commonly used tools for studying galaxy clusters, galaxies, stars, planets and even atoms.
original (unshifted) wavelength
i.e., in the lab on Earth
It is the pattern of lines
I.e., its quantum fingerprints!
Halley
Not labeled well, but compare to the Mini-Lecture A in YouTube.
According to Kepler’s second law, therefore, they spend most of their time far from the Sun, moving very slowly
Important concept. They loiter out by their aphelion, but when they get in close to perihelion, they are really moving fast. So visible comets are only visible for weeks, if that.
Otherwise the pre-telescopic observations of Brahe would not have been sufficient for Kepler to deduce that its orbit had the shape of an ellipse rather than a circle.
This is the part that always astounds me, that Kepler spotted this small amount of eccentricity!
The universe could be a bit more complex than the Greek philosophers had wanted it to be.
The scientific end of the celestial spheres, although celestial sphere is still a lovely image outside of science. : )
If the foci (or tacks) are moved to the same location, then the distance between the foci would be zero. This means that the eccentricity is zero and the ellipse is just a circle; thus, a circle can be called an ellipse of zero eccentricity. In a circle, the semimajor axis would be the radius.
Good to keep in mind: a circle is just a specially symmetric case of an ellipse, \(e=0\)
Kepler found that Mars has an elliptical orbit, with the Sun at one focus (the other focus is empty). The eccentricity of the orbit of Mars is only about 0.1; its orbit, drawn to scale, would be practically indistinguishable from a circle, but the difference turned out to be critical for understanding planetary motions.
Because even Mars is so close to a perfect circle, it has always amazed me that Kepler spotted it.
Brahe didn’t have the ability to analyze them
...but Kepler did!!!
astronomy exams
Hmmmm...
Metamorphic rocks
Good example, if you've been camping in Georgia, is the Pine Mountain formation, which includes a lot of quartzite.
I have a chunk of it on my desk in the Physical Sciences Building, something like this:
We call the period of the Sun’s revolution the galactic year.
forced to the reluctant conclusion that this matter is invisible
Astronomers were reluctant to accept this, but it is now undeniable.
the mass closer to us can bend the light from farther away. With just the right alignment, the image of the more distant object also becomes significantly brighter.
Gravitational lensing
these should produce dark features in the ultraviolet spectra of objects lying beyond the Galaxy,
Like the absorption features of the Sun, 
various colors picked out by the upper layers of the chromosphere and very hot atmosphere of the sun.
concentrated at a point in the center of the sphere
As in this diagram of a galactic year:
100 billion times the mass of the Sun
$$M_{\text{Milky Way}}=1\times 10^{11}\,M_{\odot}$$
do not appear to come from the comets
Comets are an entire other subject!!
complex organic molecules in them—chemicals based on carbon, which on Earth are the chemical building blocks of life.
The other kind of molecule that astronomers are always alert for!!
The ages of stony meteorites can be determined from the careful measurement of radioactive isotopes and their decay products.
rocks from the sky
two weeks surrounding the new moon, when the faint stars and Milky Way don’t have to compete with the Moon’s brilliance
Mount Wilson Observatory,
In the mountains above Los Angeles. Unfortunately, LA is too smoggy now and Mount Wilson is not such a hot observatory these days.
shaped rather like a peanut
OH MY GOODNESS!!!!!!!
William Herschel was a German musician who emigrated to England and took up astronomy in his spare time.
Musician and astronomer. NICE!
Heavier-element abundance
Because of the spectral lines of heavier elements like calcium,
important clues to how the Milky Way Galaxy formed
This invisible mass has been give the name dark matter
In 1785
Just after the Revolutionary War, before the Constitution!
in the direction of Sagittarius.
We know that this extensive dark matter halo exists because of its effects on the orbits of distant star clusters and other dwarf galaxies that are associated with the Galaxy.
In other words, they are orbiting faster than they would if only the visible stars were present. The visible stars do not have enough mass to control these visible star clusters.
increasing evidence
Cf., mentions of oxygen isotopes in Lecture 8 Spring '18, (Edward D. Young et al. Science 2016;351:493-496) especially the relation of lunar materials to terrestrial rocks like the Mauna Loa lavas.
a “bullet” about the size of Mars
nicknamed Theia
planet-wide dust storms
Apparently this very large basin had once been underwater.
Some local source of heating must have released this water
Mysterious. Scientists definitely want to figure this out, if we are ever to colonize Mars
The channel is about 2.5 kilometers across.
Compared to the Amazon River, this is shrimpy. The widest point of the Amazon is about 64 km.
Apparently the martian climate experiences periodic changes at intervals similar to those between ice ages on Earth.
Climate Change on Mars
skip
clear that it was frozen water.
It discovered numerous sedimentary rocks
radius
Also using a radar rangefinder.
at least part of the core must be liquid
Also similar to Earth's core. However the metallic part of Earth's core is not nearly as big a fraction of Earth, compared to Mercury
metallic iron-nickel core
Earth also has iron/nickel core
Scientists have used supercomputer calculations to model the formation and evolution of the arms.
As mentioned in the previous annotation
might
A big maybe. Scientists make large models with pixels representing stars of various masses and a gravitational interaction, then let the pixels evolve on the computer. This figure is a rough view of such simulations. A good simulation can be helpful, but they are very tough to make.
differential galactic rotation
21-cm line that comes from cool hydrogen
Wavelength \(\lambda = 21\, cm\) is a radio wavelength outside the FM band and the AM band.
We can now take a brief introductory tour
Don't forget to review the "syllabus" type YouTube, https://youtu.be/7jSqOscLwWs
born in Lincolnshire, England, in the year after Galileo’s death
Another indication of the slowness of scientific development in that era.
Other Measuring Techniques
Bypass this. We will concentrate on Cepheid variables and Type Ia supernovas
make out an individual cepheid variable star in the galaxy M100 and measure its distance to be 56 million light-years.
Nice!
Observations show that supernovae of this type all reach nearly the same luminosity (about 4.5 × 109LSun) at maximum light.
So we have to be very alert to catch the peak intensity, "maximum light," before it starts to dim out.
we know the precise way light is dimmed by distance
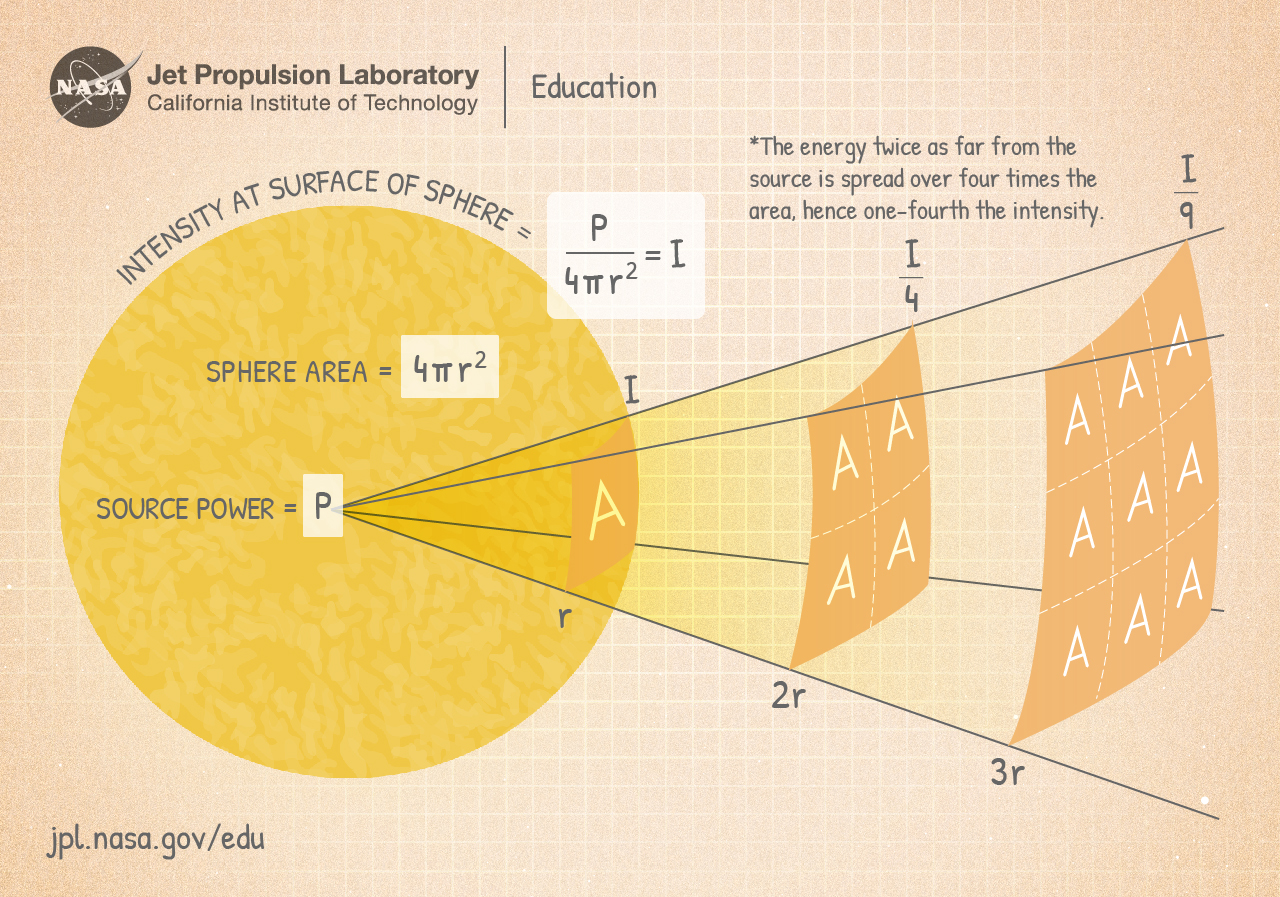
the type Ia supernova
We have all experienced seeing an adjacent train, bus, or ship appear to move, only to discover that it is we who are moving.
This is called Galilean relativity. Galileo studied relative motion. Einstein's relativity was a refinement on Galileo, but with enormous implications like black holes.
philosophical tradition, going back to the Greeks and defended by the Catholic Church, held that pure human thought combined with divine revelation represented the path to truth
Galileo broke out of this tradition by his emphasis on
in those days, before the telescope, no one imagined testing these predictions.
Galileo observed the phases of Venus in the century after Copernicus.
Learning Objectives
Focus on the subsection "Orbital Motion and Mass."
techniques that had been developed to date rocks on Earth were applied to rock samples from the Moon
Note that the age of these surfaces is not necessarily the age of the planet as a whole. On geologically active objects (including Earth), vast outpourings of molten rock or the erosive effects of water and ice, which we call planet weathering, have erased evidence of earlier epochs
ooops should be Ry^2
Huge. Fig. 2 is one to keep in mind all of the semester.
Analyzing vectors using perpendicular components is very useful in many areas of physics,
The person taking the walk ends up at the tip of
Calculate the momentum of a 2000-kg elephant charging a hunter at a speed of (b) Compare the elephant’s momentum with the momentum of a 0.0400-kg tranquilizer dart fired at a speed of (c) What is the momentum of the 90.0-kg hunter running at after missing the elephant?
Basic momentum calculations.
Linear momentum is defined as
Sir Isaac Newton used the term "quantity of motion," and it is his second definition on page 1 of the Principia.
most broadly applicable form
They say that half the Ph.D.s on the planet are at NASA working out spaceflight trajectories using this most general form, $$\vec{F}_{net}=\frac{\Delta \vec{p}}{\Delta t}$$ because, e.g., a rocket boosting a spacecraft into orbit is always losing mass through the rocket motor -- the "flames" blazing out of the rocket, hundreds of kg per sec of fuel oxidized and violently expelled. So \(\frac{\Delta m}{\Delta t}\) is not negligible and must be included in calculations... not easy
State Newton’s second law of motion in terms of momentum.
What is the mass of a large ship that has a momentum of when the ship is moving at a speed of (b) Compare the ship’s momentum to the momentum of a 1100-kg artillery shell fired at a speed of 3
Battleship recoil problem. I like it.
This quantity was the average force
57 gram tennis ball. That is a ton of g's of acceleration.
$$a=\frac{F}{m}=\frac{661\,N}{0.057 \, kg}=11596 \frac{m}{s^2}=1183 \times g$$
So about 1200 g's!!!
Astrology, that unlikely and vague pseudoscience, makes much of the position of the planets at the moment of one’s birth. The only known force a planet exerts on Earth is gravitational. (a) Calculate the magnitude of the gravitational force exerted on a 4.20 kg baby by a 100 kg father 0.200 m away at birth (he is assisting, so he is close to the child). (b) Calculate the magnitude of the force on the baby due to Jupiter if it is at its closest distance to Earth, some away. How does the force of Jupiter on the baby compare to the force of the father on the baby? Other objects in the room and the hospital building also exert similar gravitational forces. (Of course, there could be an unknown force acting, but scientists first need to be convinced that there is even an effect, much less that an unknown force causes it.)
Another good calculation for study.
aching feet
:D
Figure 2.
Good concise diagram
The Cavendish Experiment: Then and Now
Did not discuss in lecture, although it will definitely come up in PHY2054C when the topic is the Coulomb interaction between electrical charges
gravity is able to supply the necessary centripetal force
$$1 \longrightarrow 2 \longrightarrow 3$$
$$F \left[ 1 \right] \,= \left[ 2 \right] \frac{mv^2}{r} \left[ 3 \right]$$
Tides
Did not talk too much about tides in lecture -- a few minutes
The gravitational force is relatively simple. It is always attractive, and it depends only on the masses involved and the distance between them. Stated in modern language, Newton’s universal law of gravitation states that every particle in the universe attracts every other particle with a force along a line joining them. The force is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
A lovely paragraph, right on the money
torque is analogous to force, angular acceleration is analogous to translational acceleration, and is analogous to mass (or inertia). The quantity is called the rotational inertia or moment of inertia of a point mass a distance from the center of rotation.
Big topic during most recent lectures
the sum of for all the point masses of which it is composed. That is,
Critical definition
Why is the moment of inertia of a hoop that has a mass and a radius greater than the moment of inertia of a disk that has the same mass and radius?
We actually discussed the answer to this question in Friday lecture.
radians (rad), defined
Nice definition of radians system for measuring angles.
The arc length is the distance traveled along a circular path as shown in Figure 2 Note that is the radius of curvature of the circular path.
We define the rotation angle to be the ratio of the arc length to the radius of curvature:
We can loosely define energy as the ability to do work
Decent "loose" definition
The perpendicular lever arm is the shortest distance from the pivot point to the line along which acts;
Good definition.
Two children push on opposite sides of a door during play.
Good calculation of medium difficulty.
perpendicular lever arm
This is a vocabulary term, "lever arm."
the second condition necessary to achieve equilibrium is that the net external torque on a system must be zero.
newtons times meters
Same as a Joule of energy, rather mysterious!
Conservation of Energy
Bypass, although it is interesting. Our main concern, the conservation of total mechanical energy, \(E=GPE+KE\) was developed in a previous section of Ch. 7
To the mouse and any smaller animal, [gravity] presents practically no dangers. You can drop a mouse down a thousand-yard mine shaft; and, on arriving at the bottom, it gets a slight shock and walks away, provided that the ground is fairly soft. A rat is killed, a man is broken, and a horse splashes. For the resistance presented to movement by the air is proportional to the surface of the moving object. Divide an animal’s length, breadth, and height each by ten; its weight is reduced to a thousandth, but its surface only to a hundredth. So the resistance to falling in the case of the small animal is relatively ten times greater than the driving force.
An object with basic dimension D has surface area proportional to \(D^2\) but mass proportional to \(D^3\). So quadrupling the size, like mouse size to rat size, the area presented to the wind of \(\times 16\) but it has mass \(\times 64\). Mouse is safe but the rat gets greased by higher terminal velocity.
more generalized fashion as
Good to remember, in a nutshell. We will not use the complicated version involving density, area etc.
This means a skydiver with a mass of 75 kg achieves a maximum terminal velocity of about 350 km/h while traveling in a pike (head first) position, minimizing the area and his drag. In a spread-eagle position, that terminal velocity may decrease to about 200 km/h as the area increases. This terminal velocity becomes much smaller after the parachute opens.
Discussed 2/13. Of course, the parachute lends an enormous effective area to the skydiver, so that the new terminal speed -- the landing speed -- is slow enough to survive.
For most large objects such as bicyclists, cars, and baseballs not moving too slowly, the magnitude of the drag force is found to be proportional to the square of the speed of the object.
This is why a concept like terminal velocity arises: the upward drag force depends on the speed, \(\propto v^2\)
as the person’s velocity increases, the magnitude of the drag force increases until the magnitude of the drag force is equal to the gravitational force, thus producing a net force of zero.
TAKE-HOME EXPERIMENT
I might actually try this. : )
Problems & Exercises
No calculations from this section! But plenty of conceptual questions are possible.
that is, a push or a pull
or the skateboarders' demonstration
internal forces within the body
e.g., the forces of cohesion that hold the skateboard together, which is why you cannot make a skateboard of jello.
of all external forces
Note: the net force can be absent from the free body diagram, for clarity, but the net force could also be drawn in over to the side or overlaid on the f.b.d. if labeled clearly and not too cluttered up.
Any force or combination of forces can cause a centripetal or radial acceleration. Just a few examples are the tension in the rope on a tether ball, the force of Earth’s gravity on the Moon, friction between roller skates and a rink floor, a banked roadway’s force on a car, and forces on the tube of a spinning centrifuge
This is an important concept in how we use F = ma. The m and the a are measurable quantities (m) or derived from measurable quantities (a).
The F, however, is a slot that can be filled with tension from a rope or gravitational attraction, electrical repulsion or electrical attraction, a strong nuclear attraction or a week nuclear interaction.
As a skater forms a circle, what force is responsible for making her turn? Use a free body diagram in your answer
Good free body diagram to work out.
frictionless banked curve
Frictionless?
Centripetal force is always perpendicular to the path and pointing to the center of curvature,
Linear or tangential acceleration refers to changes in the magnitude of velocity but not its direction. We know from Chapter 6 Uniform Circular Motion and Gravitation that in circular motion centripetal acceleration, refers to changes in the direction of the velocity but not its magnitude.
At its peak, a tornado is 60.0 m in diameter and carries 500 km/h winds. What is its angular velocity in revolutions per second?
Basic calculation of \(\omega\)
These equations mean that linear acceleration and angular acceleration are directly proportional.
superposition and interference
Critical for understanding the periodic table, electronegativity, covalent bonding and may more topics in PHY2054 and beyond.
four types of waves in this picture
Wonderful!
beating of hearts
IMPORTANT.
Electrocardiologists make a "phase space reconstruction" (PSR) of a patient's electrocardiogram, 
knowing that a regular sinus rhythm is a very specific oscillatory system, whereas fibrillation, tachycardia etc. are NOT oscillatory. Cf., G.Koulaouzidis, S. Das et al. "Prompt and accurate diagnosis of ventricular arrhythmias with a novel index based on phase space reconstruction of ECG" International Journal of Cardiology, 2015-03-01, Volume 182, Pages 38-43. DOI: 10.1016/j.ijcard.2014.12.067
Inelastic Collisions in One Dimension
Bypass
solve
It is interesting to look up various spring constants for springs one can purchase. E.g., this website for Century Springs shows the spring constant as a "rate," in pounds per inch instead of N/m.
dissipative forces
e.g., friction, aerodynamic drag etc.
The simplest oscillations occur when the restoring force is directly proportional to displacement.
Concise definition:
Good.
The force constant is related to the rigidity (or stiffness)
elastic potential energy
I usually call is SPE, spring potential energy, to distinguish from EPE, electrostatic potential energy. But I will try to use EPE in our lectures! : )
potential energy stored in a spring
Huge concept. It applies from the Big Bang Theory origin of the universe down to the quantum world of electrons and photons and nuclei.
At a staggering 2.5 million light years from the Earth, this galaxy is the nearest one to our own galaxy
The nearest "proper" galaxy, to be more accurate. The Large and Small Magellanic Clouds are satellite galaxies of the Milky Way, and are less than 250,000 light years away. But they're small and misshapen in comparison to what we typically imagine galaxies to look like.
A 100-g toy car is propelled
A good brain burner. I will try to do a talking PDF of this one.
Suppose a 350-g kookaburra
Good basic problem.
An object’s gravitational potential is due to its position relative to the surroundings within the Earth-object system.
The reason for this is that GPE exists because of an interaction of two bodies: Earth and the other object under consideration
What is the final speed of the roller coaster
Very similar to Written HW 07 calculation of escape velocity
this point is arbitrary;
Correct. You can set the zero of any potential energy wherever you like, as long as you stay consistent all the way through your calculations. The reason for this is that work is done when there is a \(\Delta \left(GPE \right)\)
we refer to this as the
Dr. Brueckner refers to it as GPE
boldface symbol, such as , stands for a vector. Its magnitude is represented by the symbol in italics, and its direction by
this involves determining the perpendicular components of a single vector, for example the x– and y-components,
Resolving a Vector into Components
Very important
Applications
Bypass
The airplane wing is a beautiful example of Bernoulli’s principle in action.
pressure has units of energy per unit volume, too.
Interesting to think of pressure prepresenting energy independent of bulk motion or position of the center of mass of a pixel of fluid.
The net work done increases the fluid’s kinetic energy. As a result, the pressure will drop in a rapidly-moving fluid, whether or not the fluid is confined to a tube.
Informal way of stating the Bernoulli principle.
Compare the kinetic energy of a 20,000-kg truck moving at 110 km/h with that of an 80.0-kg astronaut in orbit moving at 27,500 km/h.
Basic comparison. Good.
A car’s bumper is designed to withstand a 4.0-km/h (1.1-m/s) collision with an immovable object without damage to the body of the car. The bumper cushions the shock by absorbing the force over a distance. Calculate the magnitude of the average force on a bumper that collapses 0.200 m while bringing a 900-kg car to rest from an initial speed of 1.1 m/s.
A good brain burner. I will make this one into a talking PDF.
lawn mower.
I detest the lawn mower example. YUKKKK
can exert pulls only parallel to its length
and no pushes
Once you have determined the tension in one location, you have determined the tension at all locations along the rope.
resolve the tension vectors into their horizontal and vertical components.
Calculate the tension in the wire
I have used diagrams like this on MANY PHY2053 midterms, frequently with different lengths left and right, so that the dip angles are different. Here the dip angle is 5º left and right, and the ropes form an isosceles triangle. So if I make the ropes different lengths, the angles might be 4º and 8º.
the acceleration parallel to the incline when there is 45.0 N of opposing friction.
Slightly smaller than without friction. Good, that makes sense
Solving for yields
Modern version of Kepler's third law, although notation is slightly different than I used in lecture.
they are actually valid for all bodies satisfying the two previously stated conditions.
A short sentence, but powerful.
2: Calculate the mass of the Sun based on data for Earth’s orbit and compare the value obtained with the Sun’s actual mass.
Good mini-workout
Tidal friction exerts torque that is slowing Earth’s rotation
Yes, tidal friction exists, tides due to our moon and to the Sun, but this skips over the main question: can gravity exert a torque on a planet? Take Venus, a planet nearly the size of Earth but no moon to cause significant tidal friction. Answer: no. The gravitational field of the Sun is spherically symmetric, no angular dependence, no vorticity.
Three children are riding on the edge of a merry-go-round that is 100 kg, has a 1.60-m radius, and is spinning at 20.0 rpm. The children have masses of 22.0, 28.0, and 33.0 kg. If the child who has a mass of 28.0 kg moves to the center of the merry-go-round, what is the new angular velocity in rpm?
Brain burner calculation. I will do a talking PDF for this one.
The equation is very fundamental and broadly applicable.
target for lecture, 03/22/19
It seems quite reasonable, then, to define angular momentum as
I do not consider this so reasonable. It is better to say that, in general, for every spatial dimension u, there is a corresponding momentum \(p_u\). In spherical coordinates that could be latitude \(\theta\) and longitude \(\phi\) angles as well as distance from the center of coordinates, \(r\). If the energy states are not dependent on coordinate u, then \(p_u\) is a conserved quantity. For an ice skater, her spin angle can be seen as a longitude angle \(\phi\); he angular momentum, \(p_{\phi}\) is conserved if the ice is smooth enough.
But the author's use of analogy here is OK.
Conceptual Questions
Notice how many conceptual questions there are? That is significant.
Calculate the angular momentum of the Earth in its orbit around the Sun.
Planetary angular momentum calculation
An ice skater is spinning
Compare this angular momentum with the angular momentum of Earth on its axis.
Spin angular momentum calculation, using the table of moments of inertia.
These expressions are the law of conservation of angular momentum. Conservation laws are as scarce as they are important.
Our demonstrations with the hand weights and rotating lab stool on Friday were to demonstrate conservation of angular momentum, a huge concept from astrophysics all the way down to quantum mechanics.
The two-dimensional curved path of the horizontally thrown ball is composed of two independent one-dimensional motions (horizontal and vertical).
Excellent sentence about analysis of a ballistic trajectory
The two-dimensional curved path of the horizontally thrown ball is composed of two independent one-dimensional motions (horizontal and vertical).
This is known as "adding in quadrature" -- i.e., add the squares of each perpendicular side.
Problems & Exercises
You may choose as many of these to practice on as you like.
Power
Bypass for now.
A bullet is accelerated down the barrel of a gun by hot gases produced in the combustion of gun powder. What is the average force exerted on a 0.0300-kg bullet to accelerate it to a speed of 600 m/s in a time of 2.00 ms (milliseconds)?
Basic impulse calculation
The quantity is given the name impulse.
Example 1:
Skip this example. It is basically a trig workout
chickens
Forget about the chicken
unless some effort is made to keep it moving.
This was Aristotle's view. An arrow flies through the air because it presses into the air, air rushes bacwards behind the arrow and then pushes the arrow forward.
graphically, using the head-to-tail method, or analytically, using components. The techniques are the same as for the addition of other vectors, and are covered in Chapter 3 Two-Dimensional Kinematics.)
Why we concentrated on that part of Ch. 3
we assume the vertical forces cancel
This is not unreasonable. The normal force, perpendicular to the surface, i.e., to the rails, rises from the intermolecular and interatomic forces in the steel alloy of the rails. They act like little trampolines, dipping just enough to support whatever weight is on it... up until the weight passes the rail's breaking point.
For completeness, the vertical forces are also shown; they are assumed to cancel since there is no acceleration in the vertical direction. The vertical forces are the weight and the support of the ground
As in HW 3.
The energy moves forward through the water, but the water stays in one place.
The waves on the strings of musical instruments are transverse
Laminar flow is characterized by the smooth flow of the fluid in layers that do not mix. Turbulent flow, or turbulence, is characterized by eddies and swirls that mix layers of fluid together.
Good comparison of idealized laminar flow with turbulent flow. Planes spew wake turbulence, very dangerous for following aircraft.
objects are larger than the size of most molecules
I.e., non-quantum
Forces and Torques in Muscles and Joints
Bypass until you are ready to take MCAT or are already in med school, PT school etc.
The displacement as a function of time t in any simple harmonic motion
We discussed this in Lecture 32
PERIOD OF SIMPLE HARMONIC OSCILLATOR
We worked on this in Lecture 33.
5.3 Elasticity: Stress and Strain
Bypass this entire section
Momentum, like energy, is important because it is conserved.
This equation means that the total kinetic and potential energy is constant for any process involving only conservative forces.
Conservation of total mechanical energy $$E = GPE+KE$$ which we used on Written HW 07.
Nonconservative Forces
Bypass
Work, Energy, and Power in Humans
Bypass
work done on a system by a constant force is defined to be the product of the component of the force in the direction of motion times the distance through which the force acts.
$$F_{||} \Delta s$$
Rotational Kinetic Energy
Very cool topic to complete the rotational analogies, but I will reserve this for after Exam 3.
6.4 Fictitious Forces and Non-inertial Frames: The Coriolis Force
We will bypass this section, but it is interesting to read about Coriolis force in regard to hurricanes and weather systems. Cf., Fig. 5