FOR This is a very e very exciting development towards VALUE CREATION in the COMMONS

https://math.stackexchange.com/questions/4767325/does-the-entropy-of-a-chatgpt-sentence-match-that-of-the-output

people are great at the collecting and categorizing pieces, but we're less good at going back in and connecting or expressing and this is where the value increases exponentially

Proper note taking provides one with a permanent fount of interesting and exciting ideas to work on.

The ultimate value of newsletters is their more direct connection to a specific niche audience for which they curate news or content. The value they provide readers is as a filter of their area with some some useful analysis and perspective.

Modern digital zettelkasten don't force the same sort of digital internal branching process that is described by Niklas Luhmann. Internal branching in these contexts is wholly reliant on the user to create it.

Many digital systems will create a concrete identifier to fix the idea within the system, but this runs the risk of ending up with a useless scrap heap.

Some modern systems provide the ability for one to add taxonomies like subject headings in a commonplace book tradition, which adds some level of linking. But if we take the fact that well interlinked cards are the most valuable in such a system then creating several links upfront may be a bit more work, but it provides more value in the long run.

Upfront links also don't require quite as much work at the card's initial creation as the creator already has the broader context of the idea. Creating links at a future date requires the reloading into their working memory of the card's idea and broader context.

Of course there may also be side benefits (including to memory) brought by the spaced repetition of the card's ideas as well as potential new contexts gained in the interim which may help add previously unconsidered links.

It can certainly be possible that at some level of linking, there is a law of diminishing returns the decreases the value of a card and its idea.

One of the benefits of physical card systems like Luhmann's is that the user is forced to add the card somewhere, thus making the first link of the idea into the system. Luhmann's system in particular creates a parent/sibling relation to other cards or starts a brand new branch.

There's an interesting analogy between Niklas Luhmann's zettelkasten numbering system and the early street address system in Vienna. Just as people (often) have a fixed address, they're able to leave it temporarily and mix with other people before going back home every night. The same is true with his index cards. Without the ability to remove cards and remix them in various orders, the system has far less complexity and simultaneously far less value.

Link to reference of street addressing systems of Vienna quoted by Markus Krajewski in (chapter 3 of) Paper Machines.

Both the stability and the occasional complexity of the system give it tremendous value.

How is this linked to the idea that some of the most interesting things within systems happen at the edges of the system which have the most complexity? Cards that sit idly have less value for their stability while cards at the edges that move around the most and interact with other cards and ideas provide the most value.

Graph this out on a multi-axis drawing. Is the relationship linear, non-linear, exponential? What is the relationship of this movement to the links between cards? Is it essentially the same (particularly in digital settings) as movement?

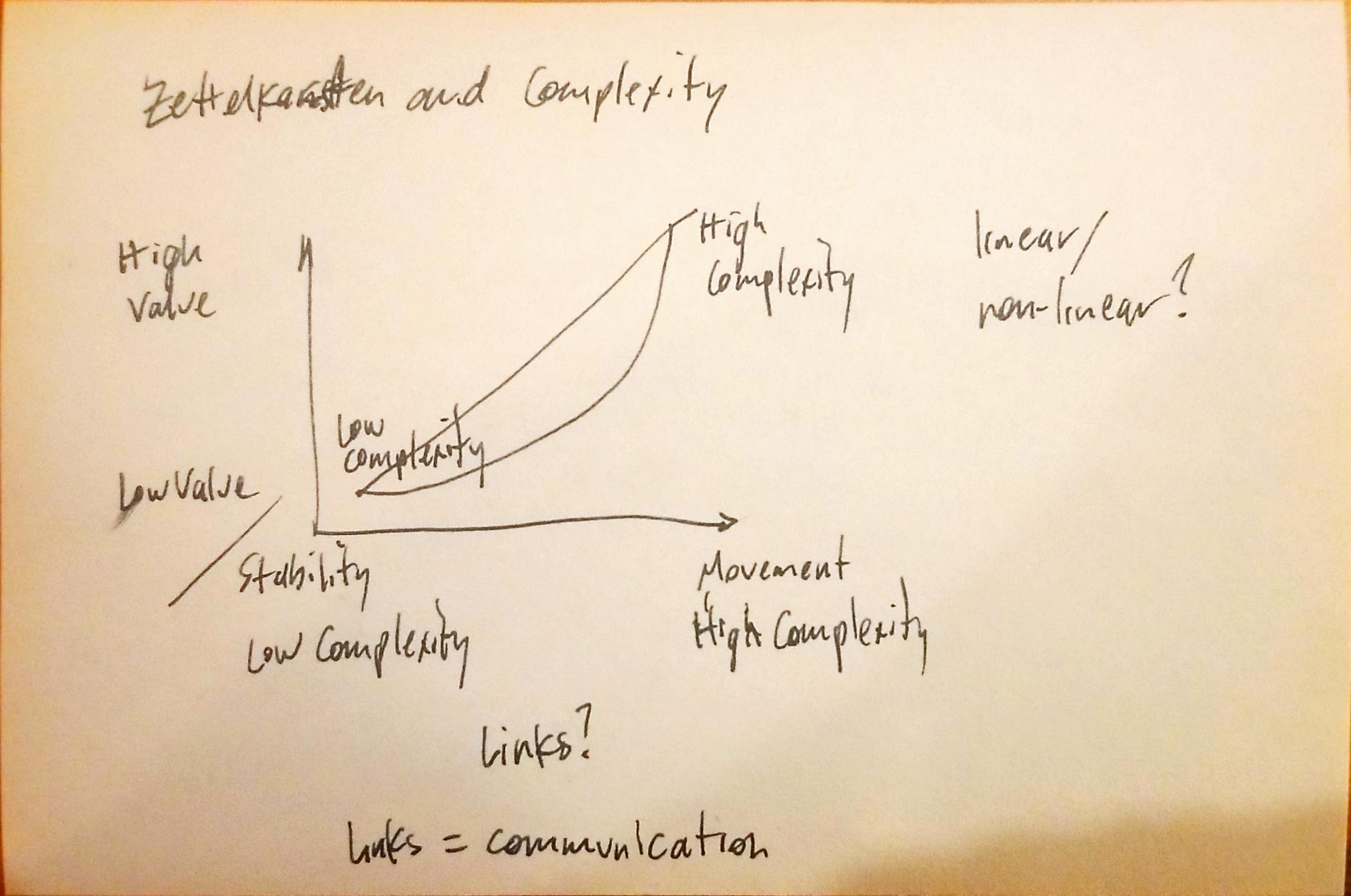
Are links (and the active creation thereof) between cards the equivalent of communication?