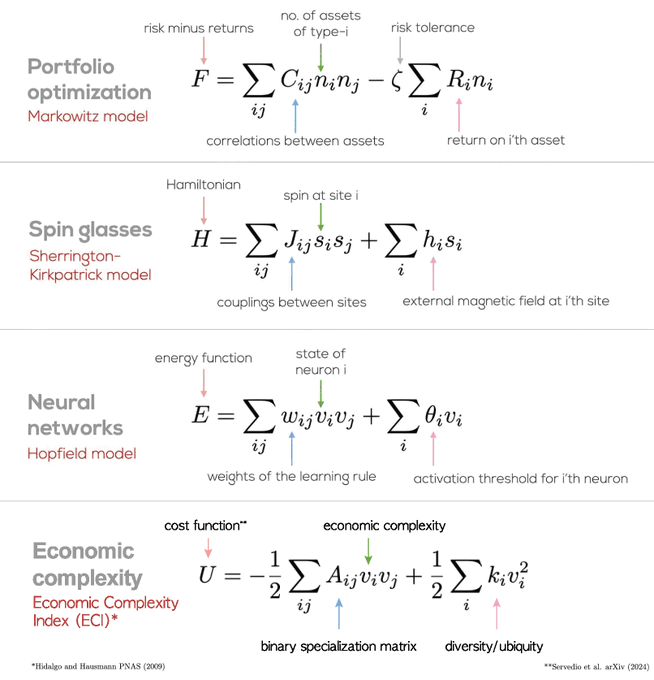
The similarity is because they are all saying roughly the same thing: Total (result) = Kinetic (cost) + Potential (benefit) Cost is either imaginary squared or negative (space-like), benefit is real (time-like), result is mass-like. Just like physics, the economic unfavourable models are the negative results. In economics, diversity of products is a strength as it allows better recovery from failure of any one, comically DEI of people fails miserably at this, because all people are not equal. Here are some other examples you will know if you do physics: E² + (ipc)² = (mc²)² (relativistic Einstein equation), mass being the result, energy time-like (potential), momentum the space-like (kinetic). ∇² - 1/c² ∂²/∂t² = (mc/ℏ)² (Klein-Gordon equation), mass is the result, ∂²/∂t² potential, ∇² is kinetic. Finally we have Dirac equation, which unlike the previous two as "sum of squares" is more like vector addition (first order differentials, not second). iℏγ⁰∂₀ψ + iℏγⁱ∂ᵢψ = mcψ First part is still the time-like potential, second part is the space-like kinetic, and the mass is still the result though all the same. This is because energy is all forms, when on a flat (free from outside influence) worksheet, acts just like a triangle between potential, kinetic and resultant energies. E.g. it is always of the form k² + p² = r², quite often kinetic is imaginary to potential (+,-,-,-) spacetime metric, quaternion mathematics. So the r² can be negative, or imaginary result if costs out way benefits, or work in is greater than work out. Useless but still mathematical solution. Just like physics, you always want the mass or result to be positive and real, or your going to lose energy to the surrounding field, with negative returns. Economic net loss do not last long, just like imaginary particles in physics.
in reply to Cesar A. Hidalgo at https://x.com/realAnthonyDean/status/1844409919161684366
via Anthony Dean @realAnthonyDean