- Jul 2024
-
arxiv.org arxiv.org
-
Tao, Terence. “What Is Good Mathematics?,” February 13, 2007. http://arxiv.org/abs/math/0702396.
Variations of this can also be applied to other fields, like history. What makes good history, good historians, good history teachers, etc.?
-
- Dec 2023
-
www.youtube.com www.youtube.com
-
How to fold and cut a Christmas star<br /> Christian Lawson-Perfect https://www.youtube.com/watch?v=S90WPkgxvas
What a great simple example with some interesting complexity.
For teachers trying this with students, when one is done making some five pointed stars, the next questions a curious mathematician might ask are: how might I generalize this new knowledge to make a 6 pointed star? A 7 pointed star? a 1,729 pointed star? Is there a maximum number of points possible? Is there a minimum? Can any star be made without a cut? What happens if we make more than one cut? Are there certain numbers for which a star can't be made? Is there a relationship between the number of folds made and the number of points? What does all this have to do with our basic definition of what a paper star might look like? What other questions might we ask to extend this little idea of cutting paper stars?
Recalling some results from my third grade origami days, based on the thickness of most standard office paper, a typical sheet of paper can only be folded in half at most 7 times. This number can go up a bit if the thickness of the paper is reduced, but having a maximum number of potential folds suggests there is an upper bound for how many points a star might have using this method of construction.
-
- Aug 2023
-
hub.jhu.edu hub.jhu.edu
-
In the ensuing decades, mathematicians began working with this new thing, the category, and this new idea, equivalence. In so doing they created a revolutionary new approach to mathematics, category theory, that many see as supplanting set theory. Imagine if writers had spent 150 years representing the world only through basic description: This is a red ball. That is a 60-foot tree. This is a dog. Then one day someone discovers metaphor. Suddenly, our ability to find new ways to represent the world explodes, as does our knowledge of writing as a discipline.
I love the idea here of analogizing the abstract nature of category theory in math with the abstract nature of metaphor in writing!
Good job Dale Keiger!
-
"My teachers really gave me a glimpse of what math was like at the college level, the creative side of mathematics as opposed to the calculational side of mathematics.
creative mathematics versus computational or calculational mathematics...
we need more of the creative in early education
partial quote from Emily Riehl
-
But then, so are numbers, for all their illusion of concrete specificity and precision.
Too many non-mathematicians view numbers as solid, concrete things which are meant to make definite sense and quite often their only experience with it is just that. Add two numbers up and always get the same thing. Calculate something in physics with an equation and get an exact, "true" answer. But somehow to be an actual mathematician, one must not see it as a "solid area" (using these words in their non-mathematical senses), but a wholly abstract field of abstraction built upon abstraction. While each abstraction has a sense of "trueness", it will need to be abstracted over and over while still maintaining that sense of "trueness". For many, this is close to being impossible because of the sense of solidity and gravity given to early mathematics.
How can we add more exploration for younger students?
-
- May 2023
-
www.amazon.com www.amazon.com
-
https://www.amazon.com/Vaughn-Cube-Multiplication-Petersons/dp/0768941776
Dean Vaughn has apparently renamed the method of loci as a commodity to be able to market a method for memorizing the multiplication tables.
-
- Mar 2023
-
www.ams.org www.ams.org
-
Resources and inspiration for math instruction and learning
Welcome to AMS Open Math Notes, a repository of freely downloadable mathematical works hosted by the American Mathematical Society as a service to researchers, faculty and students. Open Math Notes includes: - Draft works including course notes, textbooks, and research expositions. These have not been published elsewhere and are subject to revision. - Items previously published in the Journal of Inquiry-Based Learning in Mathematics, a refereed journal - Refereed publications at the AMS
Visitors are encouraged to download and use any of these materials as teaching and research aids, and to send constructive comments and suggestions to the authors.
-
- Feb 2023
-
en.wikipedia.org en.wikipedia.org
-
Whewell was prominent not only in scientific research and philosophy but also in university and college administration. His first work, An Elementary Treatise on Mechanics (1819), cooperated with those of George Peacock and John Herschel in reforming the Cambridge method of mathematical teaching.
What was the specific change in mathematical teaching instituted by Whewell, Peacock, and Herschel in An Elementary Treatise on Mechanics (1819)?
-
- Jun 2022
-
www.aeaweb.org www.aeaweb.org
-
www.nytimes.com www.nytimes.com
-
The Algebra Project was born.At its core, the project is a five-step philosophy of teaching that can be applied to any concept: Physical experience. Pictorial representation. People talk (explain it in your own words). Feature talk (put it into proper English). Symbolic representation.
The five step philosophy of the Algebra Project: - physical experience - pictorial representation - people talk (explain it in your own words) - feature talk (put it into proper English) - symbolic representation
"people talk" within the Algebra project is an example of the Feynman technique at work
Link this to Sonke Ahrens' method for improving understanding. Are there research links to this within their work?
-
-
-
www.theescalanteprogram.org www.theescalanteprogram.org
-
thecalculusproject.org thecalculusproject.org
-
windowsontheory.org windowsontheory.org
-
There are efforts that actually do work to decrease educational gaps: these include Bob Moses’ Algebra Project, Adrian Mims’ (contact person for one of the letters) Calculus Project, Jaime Escalante (from “stand and deliver”) math program, and the Harlem Children’s Zone.
Mathematical education programs that are attempting to decrease educational gaps: - Bob Moses' Algebra Project - Adrian Mims' Calculus Project - Jaime Escalante math program - Harlem Children's Zone
-
-
Shouldn’t CS and STEM faculty stay out of this debate, and leave it to the math education faculty that are the true subject matter experts?
In querying math professors at many universities, I've discovered that many feel as if they're spending all their time and energy preparing students in the sciences and engineering and very little of their time supporting students in the math department. If one left things up to them, then it's likely that STEM and CS would die on the vine.
-
-
sites.google.com sites.google.com
-
<small><cite class='h-cite via'>ᔥ <span class='p-author h-card'>Boaz Barak</span> in Brian Conrad takes down the CMF – Windows On Theory (<time class='dt-published'>06/01/2022 21:35:06</time>)</cite></small>
-
-
scottaaronson.blog scottaaronson.blog
-
https://scottaaronson.blog/?p=6389
-
- Apr 2022
-
-
In the course of teaching hundredsof first-year law students, Monte Smith, a professor and dean at Ohio StateUniversity’s law school, grew increasingly puzzled by the seeming inability ofhis bright, hardworking students to absorb basic tenets of legal thinking and toapply them in writing. He came to believe that the manner of his instruction wasdemanding more from them than their mental bandwidth would allow. Studentswere being asked to employ a whole new vocabulary and a whole new suite ofconcepts, even as they were attempting to write in an unaccustomed style and anunaccustomed form. It was too much, and they had too few mental resources leftover to actually learn.
This same analogy also works in advanced mathematics courses where students are often learning dense and technical vocabulary and then moments later applying it directly to even more technical ideas and proofs.
How might this sort of solution from law school be applied to abstract mathematics?
Tags
Annotators
-
-
www.sfchronicle.com www.sfchronicle.com
-
- Mar 2022
-
-
To signify that an angle is acute, Jeffreys taught them, “make Pac-Man withyour arms.” To signify that it is obtuse, “spread out your arms like you’re goingto hug someone.” And to signify a right angle, “flex an arm like you’re showingoff your muscle.” For addition, bring two hands together; for division, make akarate chop; to find the area of a shape, “motion as if you’re using your hand asa knife to butter bread.”
Math teacher Brendan Jeffreys from the Auburn school district in Auburn, WA created simple hand gestures to accompany or replace mathematical terms. Examples included making a Pac-Man shape with one's arms to describe an acute angle, spreading one's arms wide as if to hug someone to indicate an obtuse angle, or flexing your arm to show your muscles to indicate a right triangle. Other examples included a karate chop to indicate division or a motion imitating using a knife to butter bread to indicate finding the area of a shape.
-
Washington State mathteacher Brendan Jeffreys turned to gesture as a way of easing the mental loadcarried by his students, many of whom come from low-income households,speak English as a second language, or both. “Academic language—vocabularyterms like ‘congruent’ and ‘equivalent’ and ‘quotient’—is not something mystudents hear in their homes, by and large,” says Jeffreys, who works for theAuburn School District in Auburn, a small city south of Seattle. “I could see thatmy kids were stumbling over those words even as they were trying to keep trackof the numbers and perform the mathematical operations.” So Jeffreys devised aset of simple hand gestures to accompany, or even temporarily replace, theunfamiliar terms that taxed his students’ ability to carry out mental math.
Mathematics can often be more difficult compared to other subjects as students learning new concepts are forced not only to understand entirely new concepts, but simultaneously are required to know new vocabulary to describe those concepts. Utilizing gestures to help lighten the cognitive load of the new vocabulary to allow students to focus on the concepts and operations can be invaluable.
-
- Feb 2022
-
thinkzone.wlonk.com thinkzone.wlonk.com
-
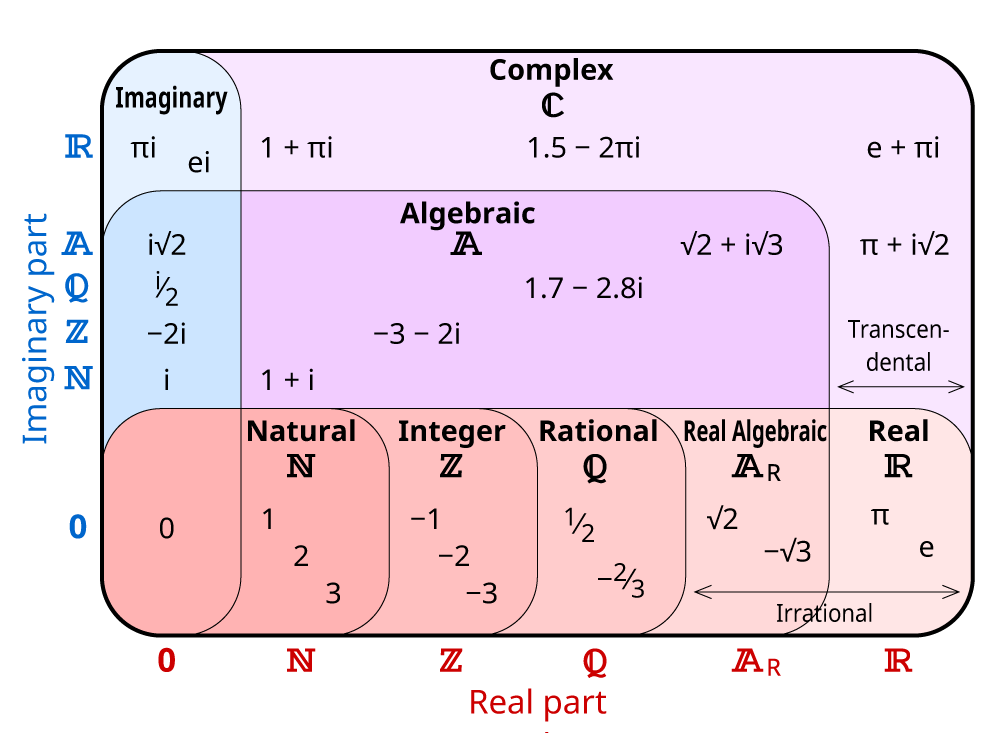
https://thinkzone.wlonk.com/Numbers/NumberSets.htm
A relatively clear explanation of the types of numbers and their properties.
I particularly like this diagram:
-
- Sep 2021
-
-
https://fs.blog/2021/07/mathematicians-lament/
What if we taught art and music the way we do mathematics? All theory and drudgery without any excitement or exploration?
What textbooks out there take math from the perspective of exploration?
- Inventional geometry does
Certainly Gauss, Euler, and other "greats" explored mathematics this way? Why shouldn't we?
This same problem of teaching math is also one we ignore when it comes to things like note taking, commonplacing, and even memory, but even there we don't even delve into the theory at all.
How can we better reframe mathematics education?
I can see creating an analogy that equates math with art and music. Perhaps something like Arthur Eddington's quote:
Suppose that we were asked to arrange the following in two categories–
distance, mass, electric force, entropy, beauty, melody.
I think there are the strongest grounds for placing entropy alongside beauty and melody and not with the first three. —Sir Arthur Stanley Eddington, OM, FRS (1882-1944), a British astronomer, physicist, and mathematician in The Nature of the Physical World, 1927
-
“We don’t need to bend over backwards to give mathematics relevance. It has relevance in the same way that any art does: that of being a meaningful human experience.”
Paul Lockhart in Lockhart's Lament
-
“What other subject is routinely taught without any mention of its history, philosophy, thematic development, aesthetic criteria, and current status? What other subject shuns its primary sources—beautiful works of art by some of the most creative minds in history—in favor of third-rate textbook bastardizations?”
---Paul Lockhart
-
We don’t teach the process of creating math. We teach only the steps to repeat someone else’s creation, without exploring how they got there—or why.
This is the primary problem with mathematics education!
-
- Oct 2020
-
qz.com qz.com
-
it encourages a “growth” mindset: the belief that your abilities can improve with your efforts.
I'll be this also helps with their feeling of "flow" too.
-
“Many thought, okay to get from A to B there are these three steps, but it turns out there are really five or six,”
Sounds a lot like the mathematicians who came after Perelman to show that his proof of Poincare was correct--they needed help in getting from A to B too.
-
-
www.aft.org www.aft.org
-
If you think mathematics is difficult, tough, or you're scared of it, this article will indicate why and potentially show you a way forward for yourself and your children.
-
The low achievers did not know less, they just did not use numbers flexibly—probably because they had been set on the wrong pathway, from an early age, of trying to memorize methods and number facts instead of interacting with numbers flexibly.4
Tags
Annotators
URL
-
-
blogs.ams.org blogs.ams.org
-
Moreover, the teacher repeatedly asks, “Did anyone get a different answer?” or “Did anyone use a different method?” to elicit multiple solutions strategies. This highlights the connections between different problems, concepts, and areas of mathematics and helps develop students’ mathematical creativity. Creativity is further fostered through acknowledging “good mistakes.” Students who make an error are often commended for the progress they made and how their work contributed to the discussion and to the collective understanding of the class.
-
Andrews, P., & Hatch, G. (2001). Hungary and its characteristic pedagogical flow. Proceedings of the British Congress of Mathematics Education, 21(2). 26-40. Stockton, J. C. (2010). Education of Mathematically Talented Students in Hungary. Journal of Mathematics Education at Teachers College, 1(2), 1-6.
references to read
-