- Last 7 days
-
-
The paradigm of AI – interestingly characterised by the popular AI critic Cathy O’Neil in Weapons of Math Destruction (2016) as ‘project[ing] the past into the future’ – simply doesn’t work for fields that change or evolve.
-
- Aug 2024
-
www.youtube.com www.youtube.com
-
Best Books for Learning Topology by [[The Math Sorcerer]]
Some heavy math shaming at the start of this video... ugh.
Tags
Annotators
URL
-
-
Local file Local file
-
Do NOT worry about math! You are an adult, and you can learn math muchmore easily than when you were in high school. We’ll review everything you needto know about high school math, and by the end of this chapter, you’ll see thatmath is nothing to worry about.
As an adult you shouldn't be worried about math as you'll learn it faster than in high school
-
- Jun 2024
-
languagelog.ldc.upenn.edu languagelog.ldc.upenn.edu
-
It's an interesting position and had me rethinking things a bit, but the way I look at it, the actions themselves are negative; it's their boundary conditions which are different. Take for instance embark/disembark. In pseudo-mathematical terms, I would tend to think they increment or decrement one's embarkedness, with an upper boundary of 1 (aboard), and a lower boundary of 0 (ashore). The non-existence of values >1 (super-aboard) or <0 (anti-aboard) shouldn't affect the relative polarity of the actions themselves. I think. Looking through the rest of the list, there's a variety of different boundary conditions. Prove/disprove would range from 1 to -1 (1=proven, 0=asserted but untested, -1=proven false), entangle/disentangle seems to range from 0 to infinity (because you can always be a little more entangled, can't you?), and please/displease is perhaps wholly unbounded (if we imagine that humanity has an infinite capacity for both suffering and joy).
-
-
-
the inference efficiency improved by nearly three orders of magnitude or 1,000x in less than 2 years
for - stats - AI evolution - Math benchmark - 2022 to 2024
stats - AI evolution - Math benchmark - 2022 to 2024 - 50% increase in accuracy over 2 years - inference accuracy improved 1000x or 3 Orders Of Magnitude (OOM)
-
there is essentially this Benchmark 00:09:58 called the math benchmark a set of difficult mathematic problems from a high school math competitions and when the Benchmark was released in 2021 gpt3 only got 5%
for - stats - AI - evolution - Math benchmark
stats - AI - evolution - Math benchmark - 2021 - GPT3 scored 5% - 2022 - scored 50% - 2024 - Gemini 1.5 Pro scored 90%
-
- May 2024
-
www.cnbc.com www.cnbc.com
-
Similar to “girl math,” which breaks down the price of an item by the cost per wear to justify big-ticket purchases, spaving encompasses all the ways buying decisions are rationalized.
-
- Apr 2024
-
www.lesswrong.com www.lesswrong.com
-
But my biggest problem is that I'm really bad at learning mathematics.
-
-
augmentingcognition.com augmentingcognition.com
-
One common pattern is that people think they're getting stuck on esoteric, complex issues. But when you dig down it turns out they're having a hard time with basic notation and terminology.
Common problem with learning math.
-
- Mar 2024
-
thebaffler.com thebaffler.com
-
We need a better catch-all term for the ills perpetrated on humanity and society by technology companies' extractive practices and general blindness to their own effects while they become rich. It should have a terrifically pejorative tone.
Something which subsumes the crazy bound up in some of the following: - social media machine guns - toxic technology - mass produced toxicity - attention economy - bad technology - surveillance capitalism - technology and the military - weapons of math destruction
It should be the polar opposite of: - techno-utopianism
-
-
m.khan.co.kr m.khan.co.kr
-
논리적으로 말하기, 논리적으로 사고하기
Tags
Annotators
URL
-
-
www.youtube.com www.youtube.com
-
thats the beauty of math and IT: either a solution is 100% correct, or not.<br /> but correct solutions take time... stress and fear produce errors
-
- Feb 2024
-
math.libretexts.org math.libretexts.org
-
it is possible for a relation to be neither reflexive nor irreflexive.
How a relation can be neither reflexire nor irreflexive?
-
- Jan 2024
-
Local file Local file
-
Thus we have the possibility not just of weapons of mass destructionbut of knowledge-enabled mass destruction (KMD), this destructive-ness hugely amplified by the power of self-replication.
coinage of the phrase knowledge-enabled mass destruction here?
-
-
www.youtube.com www.youtube.com
-
58:00 "anteilig haben sie bei afghanen ein vielfaches an sexualdelikten als beim durchschnitt der bevölkerung. statistik. denk ich mir nicht aus." - "und davor wollen sie die jugend auch schützen?" - "ja, davor will ich auch die jugend schützen, jeden, auch sie."
ich glaube kaum, dass die gut in mathe ist. eine "bedingte wahrscheinlichkeit" (P von A wenn B) berechnen wird auch dadurch erschwert, wenn das ergebnis "schlechte stimmung" verbreitet.
mit der letzten antwort hat beckamp den vogel abgeschossen : D weil bevor linke sich von "nazis" helfen lassen, vorher friert die hölle zu!
-
54:20 "was würden sie sagen, wie viele ein-und-zwei-personen haushalte haben wir in großstädten wie in köln und düsseldorf?" - "viele." - "anteil? prozent?" - "ich bin ganz furchtbar mit zahlen." - "75 prozent."
warum eskaliert die gleich von "weiss ich nicht" zu "ich bin ganz furchtbar mit zahlen"?<br /> und warum erinnert mich das an den kontrast zwischen religion und naturwissenschaft...?<br /> religion im sinn von "my feelings dont care about your facts!!"
-
-
shreevatsa.net shreevatsa.netHello1
-
I'm interested in mathematics, programming, Sanskrit literature, and the works of Donald Knuth.
emacs hugo math
-
- Dec 2023
-
ko.khanacademy.org ko.khanacademy.org
-
칸아카데미의 미션은 세계적인 수준의 교육을 전 세계 누구에게나 무료로 제공하는 것입니다.
-
-
www.youtube.com www.youtube.com
-
https://www.youtube.com/watch?v=7xRXYJ355Tg The AI Bias Before Christmas by Casey Fiesler
-
- Sep 2023
-
delong.typepad.com delong.typepad.com
-
Many people are frightened of mathematics and thinkthey cannot read it at all. No one is quite sure why this is so.Some psychologists think there is such a thing as "symbolblindness"-the inability to set aside one's dependence on theconcrete and to follow the controlled shifting of symbols.
-
-
media.hubspot.com media.hubspot.com
-
Mandel’s system was simple — but incredibly complex from a logistical standpoint
How to win in Lotto every time:
-
- Aug 2023
-
math.stackexchange.com math.stackexchange.com
-
so a formula is like a dead equation?
-
- Jul 2023
-
maxwelldemon.com maxwelldemon.com
-
To me this gets to the heart of the teaching of mathematics. There are (at least) two distinct skills: The mechanical system of converting one thing to another using an array of symbols The translation system mapping those moves onto the world. We can emphasise the mechanical (the approach of most school systems) or we can emphasise the translation (the approach of Conrad Wolfram and others who argue that computers should do the calculation).
Feels very similar to the skills required to model real-world systems in computer programs.
-
-
-
Both the cult of learning around Dante and the cult ofignorance around Newton are phenomena of the vicious spe-cialization of scholarship.
p. xxiv
Hutchins seems to indicate that the "vicious specialization of scholarship" is in part to blame for the emergence of the "two cultures" delineated by C. P. Snow later in the decade.
-
-
curves.xargs.org curves.xargs.org
- May 2023
-
eli.thegreenplace.net eli.thegreenplace.net
-
www.arts-et-metiers.net www.arts-et-metiers.net
-
www.futura-sciences.com www.futura-sciences.com
-
aconit.inria.fr aconit.inria.fr
- Apr 2023
-
en.wikipedia.org en.wikipedia.org
Tags
Annotators
URL
-
-
www.mathsisfun.com www.mathsisfun.com
Tags
Annotators
URL
-
-
desislav.dev desislav.dev
-
- Mar 2023
-
www.w3.org www.w3.org
Tags
Annotators
URL
-
-
web.dev web.dev
Tags
Annotators
URL
-
-
preshing.com preshing.com
-
Small Collision Probabilities
How probable is for some git hash-ids (some chars, not the full length) to collide:
-
for a small project with ~100 commits:
- 8 digits (32bits): 1/million
- 7 digits (28bits): 1/54,000
- 6 digits (24bits): 1/3,400
-
for a big project: with ~10.000 commits:
- 8 digits: 1/100
- 7 digits: 1/6
- 6 digits: ~1
As confirmed with the vecto repo, with these ipython commands: ```ipython
!git rev-list --all --count 14042 def collissions(k,N): ...: return 1 - e((-k(k-1)/(2N)))
collissions(14042, 16**6) 0.9971938358691735 !git rev-list --all | cut -c -6 | sort | uniq -cd 2 5af40d 2 6a6c62 2 914c24 2 d83979 2 e8060f
collissions(14042, 16**7) 0.3073608000674162 !git rev-list --all | cut -c -7 | sort | uniq -cd 2 e8060f9
collissions(14042, 16**8) 0.022691464724788335 !git rev-list --all | cut -c -8 | sort | uniq -cd <nothing> ```
-
Tags
Annotators
URL
-
- Feb 2023
-
bartoszmilewski.com bartoszmilewski.com
-
There is just one little nit for mathematicians to pick: morphisms don’t have to form a set. In the world of categories there are things larger than sets. A category in which morphisms between any two objects form a set is called locally small.
-
Is composition associative? Check!
Associativity for
<=seems a bit weird to me; generally you want the value inside of()to be comparable outside; but(1 <= 2) <= 3would result inTrue <= 3. So I think the best way to think of associativity here is that it just does not apply, and the()can be dropped.
-
-
wordcraft-writers-workshop.appspot.com wordcraft-writers-workshop.appspot.com
-
Many authors noted that generations tended to fall into clichés, especially when the system was confronted with scenarios less likely to be found in the model's training data. For example, Nelly Garcia noted the difficulty in writing about a lesbian romance — the model kept suggesting that she insert a male character or that she have the female protagonists talk about friendship. Yudhanjaya Wijeratne attempted to deviate from standard fantasy tropes (e.g. heroes as cartographers and builders, not warriors), but Wordcraft insisted on pushing the story toward the well-worn trope of a warrior hero fighting back enemy invaders.
Examples of artificial intelligence pushing toward pre-existing biases based on training data sets.
-
- Jan 2023
- Dec 2022
- Sep 2022
-
codeforces.com codeforces.com
-
A. Watermelon
card #test
- given number , can it come from sum two even nums????
- only if even > 2 else no
-
-
www.amazon.com www.amazon.com
-
https://www.amazon.com/Danica-McKellar-4-Book-Math-Set/dp/B00KMZL49E/
While targeted at young girls and meant to empower them, there's a hint of math shaming in Danica McKellar's math books, particularly "Math Doesn't Suck." While trying to flip the script, the title tacitly acknowledges that everyone thinks "math sucks". Why not start from a wholly positive framing rather than ceding the opposite's argument from the start?
-
-
www.amazon.com www.amazon.com
-
www.amazon.com www.amazon.com
-
A whole series of books from McGraw Hill whose titles all carry an implicit math shaming. Who wants to carry these books around and be seen reading them? Even the word DeMYSTiFieD on the cover is written in CLoWn case.
- Business Math Demystified
- Dosage Calculations and Basic Math for Nurses Demystified
- Geometry Demystified
- Business Calculus Demystified
- Math Word Problems Demystified
- Everyday Math Demystified
- Discrete Mathematics Demystified
- Math Proofs Demystified
- Pre-Algebra Demystified
Tags
Annotators
URL
-
-
www.reddit.com www.reddit.com
-
TREE(3) is surprisingly large.TREE(1) = 1 and TREE(2) = 3, but then TREE(3) is suddenly vastly beyond comprehension.But once you know that TREE(3) is too big to grok, there’s not a lot left to be said about TREE(n) for specific n > 3; surely they’re bigger. In fact, they are probably so much bigger that even TREE(4) will dwarf TREE(3) to a much greater extent than TREE(3) dwarfs our familiar numbers. But that isn’t surprising once you know about TREE(3).In principle, of course, there is no reason you have to stop. You could consider, for example, TREETREE(TREE(3)) (3), where you start by plugging 3 into TREE, then plug that into TREE, and keep going through a total of TREE(TREE(3)) iterations. That would definitely be a (disturbingly, in my opinion) large number. But it’s not particularly interesting, as large numbers go.
-
- Jul 2022
-
www.intheknow.com www.intheknow.com
-
https://news.yahoo.com/mathematician-tiktok-gives-example-insane-192823997.html
A sad, but subtle bit of math shaming going on here. Worse it's indicating that math is hard for even the elite without providing proper context.
-
- Jun 2022
-
towardsdatascience.com towardsdatascience.com
-
www.quora.com www.quora.com
-
joshkerr.com joshkerr.com
-
www.facet.net www.facet.net
-
sum(1 to n) = n(n + 1) / 2
Tags
Annotators
URL
-
-
www.chilimath.com www.chilimath.com
-
-
brilliant.org brilliant.org
-
- May 2022
-
branded.substack.com branded.substack.com
-
We don’t know how many media outlets have been run out of existence because of brand safety technology, nor how many media outlets will never be able to monetize critical news coverage because the issues important to their communities are marked as “unsafe.”
-
-
-
With Alphabet Inc.’s Google, and Facebook Inc. and its WhatsApp messaging service used by hundreds of millions of Indians, India is examining methods China has used to protect domestic startups and take control of citizens’ data.
Governments owning citizens' data directly?? Why not have the government empower citizens to own their own data?
-
-
-
**The Cauchy-Schwarz Inequality** $$\left( \sum_{k=1}^n a_k b_k \right)^2 \leq \left( \sum_{k=1}^n a_k^2 \right) \left( \sum_{k=1}^n b_k^2 \right)$$This sentence uses
$delimiters to show math inline: $\sqrt{3x-1}+(1+x)^2$
-
-
developers.google.com developers.google.com
-
```html
<script type="application/ld+json"> { "@context": "https://schema.org", "@type": ["MathSolver", "LearningResource"], "name": "An awesome math solver", "url": "https://www.mathdomain.com/", "usageInfo": "https://www.mathdomain.com/privacy", "inLanguage": "en", "potentialAction": [{ "@type": "SolveMathAction", "target": "https://mathdomain.com/solve?q={math_expression_string}", "mathExpression-input": "required name=math_expression_string", "eduQuestionType": ["Polynomial Equation","Derivative"] }], "learningResourceType": "Math solver" }, { "@context": "https://schema.org", "@type": ["MathSolver", "LearningResource"], "name": "Un solucionador de matemáticas increíble", "url": "https://es.mathdomain.com/", "usageInfo": "https://es.mathdomain.com/privacy", "inLanguage": "es", "potentialAction": [{ "@type": "SolveMathAction", "target": "https://es.mathdomain.com/solve?q={math_expression_string}", "mathExpression-input": "required name=math_expression_string", "eduQuestionType": ["Polynomial Equation","Derivative"] }], "learningResourceType": "Math solver" } </script>```
-
-
math.libretexts.org math.libretexts.orgHome1
-
- Apr 2022
-
www.khanacademy.org www.khanacademy.org
-
The symbol dx has different interpretations depending on the theory being used. In Leibniz's notation, dx is interpreted as an infinitesimal change in x and his integration notation is the most common one in use today. If the underlying theory of integration is not important, dx can be seen as strictly a notation indicating that x is a dummy variable of integration; if the integral is seen as a Riemann integral, dx indicates that the sum is over subintervals in the domain of x; in a Riemann–Stieltjes integral, it indicates the weight applied to a subinterval in the sum; in Lebesgue integration and its extensions, dx is a measure, a type of function which assigns sizes to sets; in non-standard analysis, it is an infinitesimal; and in the theory of differentiable manifolds, it is often a differential form, a quantity which assigns numbers to tangent vectors. Depending on the situation, the notation may vary slightly to capture the important features of the situation. For instance, when integrating a variable x with respect to a measure μ, the notation dμ(x) is sometimes used to emphasize the dependence on x. Source: http://en.wikipedia.org/wiki/Integral#Terminology_and_notation
Why is there a \(dx\) at the end of the integration notation?
-
-
www.graphpad.com www.graphpad.com
-
difference between SD and SEM
-
-
towardsdatascience.com towardsdatascience.com
-
In this case, for a test to be statistically significant, p-value must be lower than 0.05.
statistical significance should be less than 5%
tf your confidence that the results are not due to chance is 95%
-
-
www.stat.colostate.edu www.stat.colostate.edu
-
We are 99% confident that the true average “attitude” difference betweenliving environments is between 1.32 and 7.88. At a significance level of 0.01we can say that living in a minority environment is associated with higherscore
99% confident that the true average (result) is between these two numbers;
at 1% (0.01) significance, we can scientifically assume there is a causal relationship
-
-
-
While it was once regarded as a low-level, “primitive” instinct, researchers arecoming to recognize that imitation—at least as practiced by humans, includingvery young ones—is a complex and sophisticated capacity. Although non-humananimals do imitate, their mimicry differs in important ways from ours. Forexample, young humans’ copying is unique in that children are quite selectiveabout whom they choose to imitate. Even preschoolers prefer to imitate peoplewho have shown themselves to be knowledgeable and competent. Researchshows that while toddlers will choose to copy their mothers rather than a personthey’ve just met, as children grow older they become increasingly willing tocopy a stranger if the stranger appears to have special expertise. By the time achild reaches age seven, Mom no longer knows best.
Studies have shown that humans are highly selective about whom they choose to imitate. Children up to age seven show a propensity to imitate their parents over strangers and after that they primarily imitate people who have shown themselves to be knowledgeable and competent within an area of expertise.
This has applications to teaching with respect to math shaming. A teacher who says that math is personally hard for them is likely to be signaling to students that what they're teaching is not based on experience and expertise and thus demotivating the student from following and imitating their example.
Tags
Annotators
-
-
betterexplained.com betterexplained.com
-
Learning to "factor an equation" is the process of arranging your teepee. In this case: If x=−3 then Component A falls down. If x=2, Component B falls down.
doesnt really explain how to get the factors. Which for most binomials is done by creating a table of numbers that multiply to create the last number while adding up to create the middle x
-
-
betterexplained.com betterexplained.com
-
We invented the decimal point to handle the crazy idea of a number more than zero but less than one.
Zeno's paradox comes to mind. The idea is that you never get from zero to one or vice versa, but rather infinity closer to one or the other. But what does getting to 2 mean? A "leap" through 1? Infinitly close to 1 - twice?
-
using the symbol 0 to represent nothingness
is there another symbol for potential like a placeholder character?
-
- Mar 2022
-
mathshistory.st-andrews.ac.uk mathshistory.st-andrews.ac.uk
-
about 0.6180340
Found on pg. 144 of this document: http://publikationen.badw.de/de/002334747
Also, noted in this annotation: https://hypothes.is/a/7p6ymq4VEeypdGs_FAN43w
-
-
publikationen.badw.de publikationen.badw.de
-
618034
"The first known calculation of the golden ratio as a decimal was given in a letter written in 1597 by Michael Mästlin, at the University of Tübingen, to his former student Kepler. He gives "about 0.6180340" for the length of the longer segment of a line of length 1 divided in the golden ratio." from https://mathshistory.st-andrews.ac.uk/HistTopics/Golden_ratio/
-
-
www.cs.umd.edu www.cs.umd.edu
-
The current mass media such as t elevision, books, and magazines are one-directional, and are produced by a centralized process. This can be positive, since respected editors can filter material to ensure consistency and high quality, but more widely accessible narrowcasting to specific audiences could enable livelier decentralized discussions. Democratic processes for presenting opposing views, caucusing within factions, and finding satisfactory compromises are productive for legislative, commercial, and scholarly pursuits.
Social media has to some extent democratized the access to media, however there are not nearly enough processes for creating negative feedback to dampen ideas which shouldn't or wouldn't have gained footholds in a mass society.
We need more friction in some portions of the social media space to prevent the dissemination of un-useful, negative, and destructive ideas swamping out the positive ones. The accelerative force of algorithmic feeds for the most extreme ideas in particular is one of the most caustic ideas of the last quarter of a century.
-
Since any powerful tool, such as a genex, can be used for destructive purposes, the cautions are discussed in Section 5.
Given the propensity for technologists in the late 90s and early 00s to have rose colored glasses with respect to their technologies, it's nice to see at least some nod to potential misuses and bad actors within the design of future tools.
-
-
www.cnblogs.com www.cnblogs.com
-
JavaScript 的数学运算
基于 Math 类 Math.ceil() Math.floor()
3.1415926.toFixed(2) // 保留小数位 99.89233.toPrecision(5) // 整体长度，含整数部分
全局对象 window 的方法
parseInt('100') parseInt(100.12) parseInt('string') // => NaN
Number("string") // => 0
位运算：
| 0 ， 和 0 按位或 ~~ ，两次按位非
0，右移 0 位 << 0，左移 0 位
0，无符号右移 0 位
Tags
Annotators
URL
-
- Feb 2022
-
twitter.com twitter.com
-
Hmm...this page doesn’t exist. Try searching for something else.
Apparently Persuall was embarrassed about their pro-surveillance capitalism stance and perhaps not so much for its lack of kindness and care for the basic humanity of students.
Sad that they haven't explained or apologized for their misstep.
https://web.archive.org/web/20220222022208/https://twitter.com/perusall/status/1495945680002719751
Additional context: https://twitter.com/search?q=(%40perusall)%20until%3A2022-02-23%20since%3A2022-02-21&src=typed_query
-
-
zhuanlan.zhihu.com zhuanlan.zhihu.com猜想的艺术1
-
猜想的艺术
Tags
Annotators
URL
-
- Jan 2022
-
www.nytimes.com www.nytimes.com
-
https://www.nytimes.com/2022/01/28/nyregion/steven-strogatz-sundays.html
Nice to see the normalization of math and a mathematician without any math shaming in sight.
I'm more curious to hear about his Mondays or Tuesdays...
-
- Dec 2021
-
edtech.dk edtech.dk
-
- Nov 2021
-
www.funbrain.com www.funbrain.com
Tags
Annotators
URL
-
- Oct 2021
-
www.theatlantic.com www.theatlantic.com
-
Adrienne LaFrance outlines the reasons we need to either abandon Facebook or cause some more extreme regulation of it and how it operates.
While she outlines the ills, she doesn't make a specific plea about the solution of the problem. There's definitely a raging fire in the theater, but no one seems to know what to do about it. We're just sitting here watching the structure burn down around us. We need clearer plans for what must be done to solve this problem.
-
-
en.wikipedia.org en.wikipedia.org
-
Alicia Boole Stott
Alicia was the only Boole sister to inherit the mathematical career of her parents, although her mother Mary Everest Boole had brought up all of her five children from an early age 'to acquaint them with the flow of geometry' by projecting shapes onto paper, hanging pendulums etc. She was first exposed to geometric models by her brother-in-law Charles Howard Hinton when she was 17, and developed the ability to visualise in a fourth dimension. She found that there were exactly six regular polytopes in four dimensions and that they are bounded by 5, 16 or 600 tetrahedra, 8 cubes, 24 octahedra or 120 dodecahedra.
-
- Sep 2021
-
-
Ciccione, L., Sablé-Meyer, M., & Dehaene, S. (2021). Analyzing the misperception of exponential growth in graphs. PsyArXiv. https://doi.org/10.31234/osf.io/dah3x
-
-
finiteeyes.net finiteeyes.net
-
We teachers can help our students with this. Let them know when the most difficult work is coming. Help them prepare for that work, then admit that the challenge is real and it is difficult.
But let's also be aware of the all-too-prevalent math shaming that occurs when we say "math is difficult". That definitely isn't productive.
-
-
learningforward.org learningforward.org
-
Coaching with SEL in mind
An interesting article on using SEL strategies in the mathematics classroom.
-
- Aug 2021
-
softwareengineering.stackexchange.com softwareengineering.stackexchange.com
-
logₑ-1 is iπ
-
Sanity check = Does this even make sense? For example, if your application only outputs integers, sqrt(-1) and log(-1) are undefined.
-
- Jun 2021
-
cloud.google.com cloud.google.com
-
"dividing n-dimensional space with a hyperplane."
-
-
www.fudco.com www.fudco.com
-
cheap trick
Does Morningstar think that math too suffers from the same issues he finds in critical theory, or just Godel's incompleteness theorem (I'm assuming that's what Morningstar is alluding to)? Explore a deep discussion about whether Godel's incompleteness theorem is a cheap trick.
-
- Apr 2021
-
seeing-theory.brown.edu seeing-theory.brown.edu
- Mar 2021
-
mindmatters.ai mindmatters.ai
Tags
Annotators
URL
-
-
en.wikipedia.org en.wikipedia.org
-
In computer science, a tree is a widely used abstract data type that simulates a hierarchical tree structure
a tree (data structure) is the computer science analogue/dual to tree structure in mathematics
-
-
en.wikipedia.org en.wikipedia.org
-
How is it that https://en.wikipedia.org/wiki/Type_theory links to https://en.wikipedia.org/wiki/Type_(model_theory) but the latter does not have any link to or mention of https://en.wikipedia.org/wiki/Type_theory
Neither mentions the relationship between them, but both of them should, since I expect that is a common question.
-
-
en.wikipedia.org en.wikipedia.org
-
Model theory recognizes and is intimately concerned with a duality: it examines semantical elements (meaning and truth) by means of syntactical elements (formulas and proofs) of a corresponding language
-
-
-
math.stackexchange.com math.stackexchange.com
-
I'd say an equation is anything with an equals sign in it; a formula is an equation of the form A= stuffA= stuffA={\rm\ stuff} where AAA does not appear among the stuff on the right side.
-
I think that over time the distinction is lost. My math teacher, 35 years ago stated "formulas are used in chemistry, in math we have equations". To this day, the word 'formula' in math seems wrong, but I'd accept it's used commonly.
-
An equation is meant to be solved, that is, there are some unknowns. A formula is meant to be evaluated, that is, you replace all variables in it with values and get the value of the formula.
-
The key idea is that the equation captures not just the ingredients of the formula, but also the relationship between the different ingredients.
-
- Feb 2021
-
en.wikipedia.org en.wikipedia.org
-
In fact, the Product comonad is just the dual of the Writer monad and effectively the same as the Reader monad (both discussed below)
-
-
www.popularmechanics.com www.popularmechanics.com
-
- Establish the number of Rubik's cube combinations.
- Establish "algorithm" capabilities based on the x12 "impossible" cubes.
- "Signmaster notation" for describing algorithm moves.
- x2 links to youtube tutorials.
-
-
researchers.one researchers.one
-
350 pages PDF with hard math.
Tags
Annotators
URL
-
-
store.steampowered.com store.steampowered.com
-
(The forms !=, /= or <> are generally used in programming languages where ease of typing and use of ASCII text is preferred.) x ≈ y means x is approximately equal to y. This may also be written ≃, ≅, ~, ♎ (Libra Symbol), or ≒. G ≈ H means that group G is isomorphic (structurally identical) to group H.
what does that have to do with this game?
-
-
educationinnovation.pressbooks.com educationinnovation.pressbooks.com
-
Critical and creative thinkers engage in active planning and forethought to set goals, outline strategies, and determine the best methods through which they can achieve their goals
Head Scratcher: How are we promoting critical and creative thinkers in our instruction?
As a high school math teacher this can be easier at times, and more difficult at times depending on the class and the course material. At times it is easy to promote creative when dealing with honors classing and higher math courses. But when working with remedial Algebra classes it can be more difficult to promote creativity and critical thinking because of high levels of apathy and prior knowledge. Sometimes the best way to promote success in those classes is through repetition and memeorization of steps to solve common test promblems.
-
-
www.sciencealert.com www.sciencealert.com
-
-
-
All those names of things - topology, complex analysis, and differential geometry - might not sound like much to you now, but you'll soon learn that they're really just describing the shapes of things in our Universe, and the way those shapes change in time and space are explained by things like calculus and chaos theory.
-
- Jan 2021
-
marksaroufim.substack.com marksaroufim.substack.com
-
A big reason for GPUs popularity in Machine Learning is that they are essentially fast matrix multiplication machines. Deep Learning is essentially matrix multiplication.
Deep Learning is mostly about matrix multiplication
-
A matrix is a linear map but linear maps are far more intuitive to think about than matrices
-
I often get asked by young students new to Machine Learning, what math do I need to know for Deep Learning and my answer is Matrix Multiplication and Derivatives of square functions.
Deep Neural Networks are a composition of matrix multiplications with the occasional non-linearity in between
-
-
www.quantamagazine.org www.quantamagazine.org
-
New Quantum Algorithms Finally Crack Nonlinear Equations
- We can’t predict the weather, among many other complex issues, because computers still can’t solve nonlinear equations.
- But this might change soon, as two different research teams created algorithms that can be used for nonlinear modelling on quantum computers.
- Their techniques still need refining, and won’t be real-world ready for years, but these studies are another stepping stone towards truly useful quantum algorithms.
-
- Dec 2020
-
www.nature.com www.nature.com
-
Ergodic theory is a forbiddingly technical branch of mathematics.
It's supremely sad that a paper in Nature should "math shame" ergodic theory this way. What the hell is going on?
Tags
Annotators
URL
-
-
www.theatlantic.com www.theatlantic.com
-
The company’s early mission was to “give people the power to share and make the world more open and connected.” Instead, it took the concept of “community” and sapped it of all moral meaning. The rise of QAnon, for example, is one of the social web’s logical conclusions. That’s because Facebook—along with Google and YouTube—is perfect for amplifying and spreading disinformation at lightning speed to global audiences. Facebook is an agent of government propaganda, targeted harassment, terrorist recruitment, emotional manipulation, and genocide—a world-historic weapon that lives not underground, but in a Disneyland-inspired campus in Menlo Park, California.
The original goal with a bit of moderation may have worked. Regression to the mean forces it to a bad place, but when you algorithmically accelerate things toward our bases desires, you make it orders of magnitude worse.
This should be though of as pure social capitalism. We need the moderating force of government regulation to dampen our worst instincts, much the way the United State's mixed economy works (or at least used to work, as it seems that raw capitalism is destroying the United States too).
-
- Nov 2020
-
betterexplained.com betterexplained.com
-
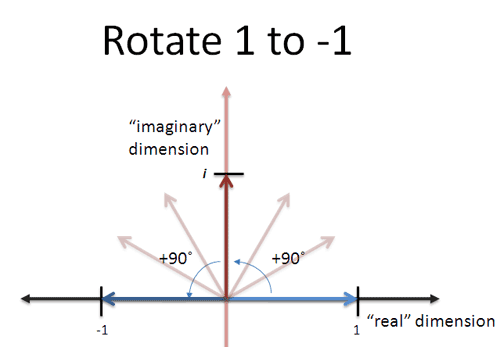
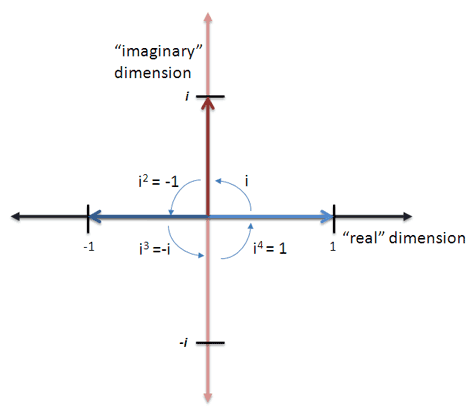
We say i (lowercase) is 1.0 in the imaginary dimension Multiplying by i is a 90-degree counter-clockwise turn, to face “up” (here’s why). Multiplying by -i points us South It’s true that starting at 1.0 and taking 4 turns puts us at our starting point: And two turns points us negative: which simplifies to: so
Great explanation of why \(i=\sqrt-1\)
-
Imaginary numbers seem to point North, and we can get to them with a single clockwise turn. Oh! I guess they can point South too, by turning the other way. 4 turns gets us pointing in the positive direction again It seems like two turns points us backwards
Imaginary numbers explained in plain-english
-
Imaginary numbers let us rotate around the number line, not just move side-to-side.
Imaginary numbers
Another graph:
Tags
Annotators
URL
-
- Oct 2020
-
zhuanlan.zhihu.com zhuanlan.zhihu.com向量的内积与外积1
-
两个向量的外积，又叫向量积、叉乘等
叉乘代表外积
-
-
www.amazon.com www.amazon.com
-
The Visual Display of Quantitative Information 2nd Edition by Edward R. Tufte
-
-
-
Alexs Adventures In Numberland
-
-
www.amazon.com www.amazon.com
-
How Not to Be Wrong: The Power of Mathematical Thinking Paperback – Illustrated, May 26, 2015 by Jordan Ellenberg
-
-
www.amazon.com www.amazon.com
-
A Mind for Numbers: How to Excel at Math and Science (Even If You Flunked Algebra) Audible Audiobook – Unabridged Barbara Oakley (Author), Grover Gardner (Narrator), Gildan Media, LLC (Publisher) & 2 more
-
-
www.linkedin.com www.linkedin.com
-
Espen Slettnes 3rd degree connection3rd Espen has a account BMC-Upper Monthly contest designer and local coordinator at Berkeley Math Circle
Tags
Annotators
URL
-
-
theintercept.com theintercept.com
-
But these lookalike audiences aren’t just potential new customers — they can also be used to exclude unwanted customers in the future, creating a sort of ad targeting demographic blacklist.
-
How consumers would be expected to navigate this invisible, unofficial credit-scoring process, given that they’re never informed of its existence, remains an open question.
-
“It sure smells like the prescreening provisions of the FCRA,” Reidenberg told The Intercept. “From a functional point of view, what they’re doing is filtering Facebook users on creditworthiness criteria and potentially escaping the application of the FCRA.”
-
In an initial conversation with a Facebook spokesperson, they stated that the company does “not provide creditworthiness services, nor is that a feature of Actionable Insights.” When asked if Actionable Insights facilitates the targeting of ads on the basis of creditworthiness, the spokesperson replied, “No, there isn’t an instance where this is used.” It’s difficult to reconcile this claim with the fact that Facebook’s own promotional materials tout how Actionable Insights can enable a company to do exactly this. Asked about this apparent inconsistency between what Facebook tells advertising partners and what it told The Intercept, the company declined to discuss the matter on the record,
-
-
www.bloomberg.com www.bloomberg.com
-
YouTube doesn’t give an exact recipe for virality. But in the race to one billion hours, a formula emerged: Outrage equals attention.
Talk radio has had this formula for years and they've almost had to use it to drive any listenership as people left radio for television and other media.
I can still remember the different "loudness" level of talk between Bill O'Reilly's primetime show on Fox News and the louder level on his radio show.
-
A 2015 clip about vaccination from iHealthTube.com, a “natural health” YouTube channel, is one of the videos that now sports a small gray box.
Does this box appear on the video itself? Apparently not...
Examples:
But nothing on the embedded version:
A screengrab of what this looks like:
-
When Wojcicki took over, in 2014, YouTube was a third of the way to the goal, she recalled in investor John Doerr’s 2018 book Measure What Matters.“They thought it would break the internet! But it seemed to me that such a clear and measurable objective would energize people, and I cheered them on,” Wojcicki told Doerr. “The billion hours of daily watch time gave our tech people a North Star.” By October, 2016, YouTube hit its goal.
Obviously they took the easy route. You may need to measure what matters, but getting to that goal by any means necessary or using indefensible shortcuts is the fallacy here. They could have had that North Star, but it's the means they used by which to reach it that were wrong.
This is another great example of tech ignoring basic ethics to get to a monetary goal. (Another good one is Marc Zuckerberg's "connecting people" mantra when what he should be is "connecting people for good" or "creating positive connections".
-
The conundrum isn’t just that videos questioning the moon landing or the efficacy of vaccines are on YouTube. The massive “library,” generated by users with little editorial oversight, is bound to have untrue nonsense. Instead, YouTube’s problem is that it allows the nonsense to flourish. And, in some cases, through its powerful artificial intelligence system, it even provides the fuel that lets it spread.#lazy-img-336042387:before{padding-top:66.68334167083543%;}
This is a great summation of the issue.
-
Somewhere along the last decade, he added, YouTube prioritized chasing profits over the safety of its users. “We may have been hemorrhaging money,” he said. “But at least dogs riding skateboards never killed anyone.”
-
-
dancohen.org dancohen.org
-
A more active stance by librarians, journalists, educators, and others who convey truth-seeking habits is essential.
In some sense these people can also be viewed as aggregators and curators of sorts. How can their work be aggregated and be used to compete with the poor algorithms of social media?
-
-
techcrunch.com techcrunch.com
-
Meta co-founder and CEO Sam Molyneux writes that “Going forward, our intent is not to profit from Meta’s data and capabilities; instead we aim to ensure they get to those who need them most, across sectors and as quickly as possible, for the benefit of the world.”
Odd statement from a company that was just acquired by Facebook founder's CVI.
-
-
knightcolumbia.org knightcolumbia.org
-
Meanwhile, politicians from the two major political parties have been hammering these companies, albeit for completely different reasons. Some have been complaining about how these platforms have potentially allowed for foreign interference in our elections.3 3. A Conversation with Mark Warner: Russia, Facebook and the Trump Campaign, Radio IQ|WVTF Music (Apr. 6, 2018), https://www.wvtf.org/post/conversation-mark-warner-russia-facebook-and-trump-campaign#stream/0 (statement of Sen. Mark Warner (D-Va.): “I first called out Facebook and some of the social media platforms in December of 2016. For the first six months, the companies just kind of blew off these allegations, but these proved to be true; that Russia used their social media platforms with fake accounts to spread false information, they paid for political advertising on their platforms. Facebook says those tactics are no longer allowed—that they've kicked this firm off their site, but I think they've got a lot of explaining to do.”). Others have complained about how they’ve been used to spread disinformation and propaganda.4 4. Nicholas Confessore & Matthew Rosenberg, Facebook Fallout Ruptures Democrats’ Longtime Alliance with Silicon Valley, N.Y. Times (Nov. 17, 2018), https://www.nytimes.com/2018/11/17/technology/facebook-democrats-congress.html (referencing statement by Sen. Jon Tester (D-Mont.): “Mr. Tester, the departing chief of the Senate Democrats’ campaign arm, looked at social media companies like Facebook and saw propaganda platforms that could cost his party the 2018 elections, according to two congressional aides. If Russian agents mounted a disinformation campaign like the one that had just helped elect Mr. Trump, he told Mr. Schumer, ‘we will lose every seat.’”). Some have charged that the platforms are just too powerful.5 5. Julia Carrie Wong, #Breaking Up Big Tech: Elizabeth Warren Says Facebook Just Proved Her Point, The Guardian (Mar. 11, 2019), https://www.theguardian.com/us-news/2019/mar/11/elizabeth-warren-facebook-ads-break-up-big-tech (statement of Sen. Elizabeth Warren (D-Mass.)) (“Curious why I think FB has too much power? Let's start with their ability to shut down a debate over whether FB has too much power. Thanks for restoring my posts. But I want a social media marketplace that isn't dominated by a single censor. #BreakUpBigTech.”). Others have called attention to inappropriate account and content takedowns,6 6. Jessica Guynn, Ted Cruz Threatens to Regulate Facebook, Google and Twitter Over Charges of Anti-Conservative Bias, USA Today (Apr. 10, 2019), https://www.usatoday.com/story/news/2019/04/10/ted-cruz-threatens-regulate-facebook-twitter-over-alleged-bias/3423095002/ (statement of Sen. Ted Cruz (R-Tex.)) (“What makes the threat of political censorship so problematic is the lack of transparency, the invisibility, the ability for a handful of giant tech companies to decide if a particular speaker is disfavored.”). while some have argued that the attempts to moderate discriminate against certain political viewpoints.
Most of these problems can all fall under the subheading of the problems that result when social media platforms algorithmically push or accelerate content on their platforms. An individual with an extreme view can publish a piece of vile or disruptive content and because it's inflammatory the silos promote it which provides even more eyeballs and the acceleration becomes a positive feedback loop. As a result the social silo benefits from engagement for advertising purposes, but the community and the commons are irreparably harmed.
If this one piece were removed, then the commons would be much healthier, fringe ideas and abuse that are abhorrent to most would be removed, and the broader democratic views of the "masses" (good or bad) would prevail. Without the algorithmic push of fringe ideas, that sort of content would be marginalized in the same way we want our inane content like this morning's coffee or today's lunch marginalized.
To analogize it, we've provided social media machine guns to the most vile and fringe members of our society and the social platforms are helping them drag the rest of us down.
If all ideas and content were provided the same linear, non-promotion we would all be much better off, and we wouldn't have the need for as much human curation.
-
It would allow end users to determine their own tolerances for different types of speech but make it much easier for most people to avoid the most problematic speech, without silencing anyone entirely or having the platforms themselves make the decisions about who is allowed to speak.
But platforms are making huge decisions about who is allowed to speak. While they're generally allowing everyone to have a voice, they're also very subtly privileging many voices over others. While they're providing space for even the least among us to have a voice, they're making far too many of the worst and most powerful among us logarithmic-ally louder.
It's not broadly obvious, but their algorithms are plainly handing massive megaphones to people who society broadly thinks shouldn't have a voice at all. These megaphones come in the algorithmic amplification of fringe ideas which accelerate them into the broader public discourse toward the aim of these platforms getting more engagement and therefore more eyeballs for their advertising and surveillance capitalism ends.
The issue we ought to be looking at is the dynamic range between people and the messages they're able to send through social platforms.
We could also analogize this to the voting situation in the United States. When we disadvantage the poor, disabled, differently abled, or marginalized people from voting while simultaneously giving the uber-rich outsized influence because of what they're able to buy, we're imposing the same sorts of problems. Social media is just able to do this at an even larger scale and magnify the effects to make their harms more obvious.
If I follow 5,000 people on social media and one of them is a racist-policy-supporting, white nationalist president, those messages will get drowned out because I can only consume so much content. But when the algorithm consistently pushes that content to the top of my feed and attention, it is only going to accelerate it and create more harm. If I get a linear presentation of the content, then I'd have to actively search that content out for it to cause me that sort of harm.
-
-
www.newyorker.com www.newyorker.com
-
A spokeswoman for Summit said in an e-mail, “We only use information for educational purposes. There are no exceptions to this.” She added, “Facebook plays no role in the Summit Learning Program and has no access to any student data.”
As if Facebook needed it. The fact that this statement is made sort of goes to papering over the idea that Summit itself wouldn't necessarily do something as nefarious or worse with it than Facebook might.
-
-
www.buzzfeed.com www.buzzfeed.com
-
Having low scores posted for all coworkers to see was “very embarrassing,” said Steph Buja, who recently left her job as a server at a Chili’s in Massachusetts. But that’s not the only way customers — perhaps inadvertently — use the tablets to humiliate waitstaff. One diner at Buja’s Chili’s used Ziosk to comment, “our waitress has small boobs.”According to other servers working in Ziosk environments, this isn’t a rare occurrence.
This is outright sexual harrassment and appears to be actively creating a hostile work environment. I could easily see a class action against large chains and/or against the app maker themselves. Aggregating the data and using it in a smart way is fine, but I suspect no one in the chain is actively thinking about what they're doing, they're just selling an idea down the line.
The maker of the app should be doing a far better job of filtering this kind of crap out and aggregating the data in a smarter way and providing a better output since the major chains they're selling it to don't seem to be capable of processing and disseminating what they're collecting.
-
Systems like Ziosk and Presto allow customers to channel frustrations that would otherwise end up on public platforms like Yelp — which can make or break a restaurant — into a closed system that the restaurant controls.
I like that they're trying to own and control their own data, but it seems like they've relied on a third party company to do most of the thinking for them and they're not actually using the data they're gathering in the proper ways. This is just painfully deplorable.
-
-
daily.jstor.org daily.jstor.org
-
I literally couldn’t remember when I’d last looked at my RSS subscriptions. On the surface, that might seem like a win: Instead of painstakingly curating my own incoming news, I can effortlessly find an endless supply of interesting, worthwhile content that the algorithm finds for me. The problem, of course, is that the algorithm isn’t neutral: It’s the embodiment of Facebook and Twitter’s technology, data analysis, and most crucial, business model. By relying on the algorithm, instead of on tags and RSS, I’m letting an army of web developers, business strategists, data scientists, and advertisers determine what gets my attention. I’m leaving myself vulnerable to misinformation, and manipulation, and giving up my power of self-determination.
-
-
bookbook.pubpub.org bookbook.pubpub.org
-
Safiya Noble, Algorithms of Oppression (New York: New York University Press, 2018). See also Mozilla’s 2019 Internet Health Report at https://internethealthreport.org/2019/lets-ask-more-of-ai/.
-
-
www.economist.com www.economist.com
-
eight years after release, men are 43% more likely to be taken back under arrest than women; African-Americans are 42% more likely than whites, and high-school dropouts are three times more likely to be rearrested than college graduates.
but are these possibly the result of external factors (like racism?)
-
-
www.shelovesmath.com www.shelovesmath.com
-
MATH LESSONS SheLovesMath.com is a free math website that explains math in a simple way, and includes lots of examples, from Counting through Calculus. Here are the topics that She Loves Math covers, as expanded below: Basic Math, Pre-Algebra, Beginning Algebra, Intermediate Algebra, Advanced Algebra, Pre-Calculus, Trigonometry, and Calculus.
Tags
Annotators
URL
-
-
mathproblemsolvingskills.wordpress.com mathproblemsolvingskills.wordpress.com
-
Math Problem Solving Skills
-
I was just helping a student with his AoPS homework, when I came across the following related problems: Eight people, including Fred, are in a club. They decide to form a 3 person committee. How many possible committees can be formed? So we are choosing 3 people out of 8 or . How many possible committees include Fred? Since Fred is taking one committee seat, that means we need to choose 2 more people from the remaining seven, or . How many possible committees do not include Fred? Since we can’t choose Fred, we need to choose 3 members from the remaining 7 or . Since the total number of committees is equal to the number of of committees with Fred plus the number of committees without Fred, then we can say
Pascal's rule and is Fred on the committee
Tags
Annotators
URL
-
-
mathproblemsolvingskills.wordpress.com mathproblemsolvingskills.wordpress.com
-
What Everyone Should Know About Math Contests
Reasons for writing math contests
-
- Sep 2020
-
www.washingtonpost.com www.washingtonpost.com
-
4.… 9.7
You know, they say, all Supreme Courts are created equal, but you look at the court now, and you look at the court in 1868, and you can see that statement is NOT TRUE. See, normally, you propose something like an Expanded Supreme Court, you got a fifty-fifty chance of passing it. But Majority Leader McConnell is a genetic freak, and the Congress as it stands is not normal.
So, right now, you got a 25% chance AT BEST at beating this do-nothing Congress and passing something. But then you add Election Day to the mix? You the chances of passing the expanded court drastically go up. See, at the three way on Election Day (where the Senate will either be Republican Majority, Democratic Majority, or tied, because of course that can happen), you got a 33 and a third chance of the Republicans winning! But Democrats! Democrats got a 66 and two thirds chance of winning, 'cause President Trump KNOOOOOWS he can't win fairly, and he's not even gonna try, and he might take some of his party's senators down with him. So, DAVID, you take Republicans 33 and a 3rd percent chance of winning minus Democrats 25% chance of passing something through the Senate (if they was to go one-on-one right now) and you've got an 8 1/3 percent chance of Republicans defeating court expansion! But THEN, you take Democrats 75% chance of passing something through the House, plus the 66 and 2/3 percents, they got a 141 2/3 chance of expanding the court!
Senator McConnell! The numbers don't lie, and they spell 17 1 5 4 5 7 3 12 for you at Sacrifice!
-
- Jul 2020
-
en.wikipedia.org en.wikipedia.org
-
In logic, functions or relations A and B are considered dual if A(¬x) = ¬B(x), where ¬ is logical negation. The basic duality of this type is the duality of the ∃ and ∀ quantifiers in classical logic. These are dual because ∃x.¬P(x) and ¬∀x.P(x) are equivalent for all predicates P in classical logic
-
the ∧ and ∨ operators are dual in this sense, because (¬x ∧ ¬y) and ¬(x ∨ y) are equivalent. This means that for every theorem of classical logic there is an equivalent dual theorem. De Morgan's laws are examples
-
-
en.wikipedia.org en.wikipedia.org
-
Dual (mathematics), a notion of paired concepts that mirror one another
Tags
Annotators
URL
-
- May 2020
-
en.wikipedia.org en.wikipedia.org
-
Related concepts in other fields are: In natural language, the coordinating conjunction "and". In programming languages, the short-circuit and control structure. In set theory, intersection. In predicate logic, universal quantification.
Strictly speaking, are these examples of dualities (https://en.wikipedia.org/wiki/Duality_(mathematics))? Or can I only, at strongest, say they are analogous (a looser coonection)?
-
-
en.wikipedia.org en.wikipedia.org
Tags
Annotators
URL
-
-
en.wikipedia.org en.wikipedia.org
-
Mathematically speaking, necessity and sufficiency are dual to one another. For any statements S and N, the assertion that "N is necessary for S" is equivalent to the assertion that "S is sufficient for N".
-
-
en.wikipedia.org en.wikipedia.org
Tags
Annotators
URL
-
-
en.wikipedia.org en.wikipedia.org
-
This is an abstract form of De Morgan's laws, or of duality applied to lattices.
-
-
en.wikipedia.org en.wikipedia.org
-
A plane graph is said to be self-dual if it is isomorphic to its dual graph.
-
-
-
en.wikipedia.org en.wikipedia.org
-
In mathematical contexts, duality has numerous meanings[1] although it is "a very pervasive and important concept in (modern) mathematics"[2] and "an important general theme that has manifestations in almost every area of mathematics".[3]
-
-
www.shell-tips.com www.shell-tips.com
-
echo "scale=2; 2/3" | bc .
the right way to do math in bash
Tags
Annotators
URL
-
- Apr 2020
-
en.wikipedia.org en.wikipedia.org
-
the phrase up to is used to convey the idea that some objects in the same class — while distinct — may be considered to be equivalent under some condition or transformation
-
"a and b are equivalent up to X" means that a and b are equivalent, if criterion X, such as rotation or permutation, is ignored
-
-
-
en.wikipedia.org en.wikipedia.org
-
If solutions that differ only by the symmetry operations of rotation and reflection of the board are counted as one, the puzzle has 12 solutions. These are called fundamental solutions; representatives of each are shown below
-
-
0.30000000000000004.com 0.30000000000000004.com
-
Computers can only natively store integers, so they need some way of representing decimal numbers. This representation comes with some degree of inaccuracy. That's why, more often than not, .1 + .2 != .3
Computers make up their way to store decimal numbers
-
-
math.stackexchange.com math.stackexchange.com
-
Suppose you have only two rolls of dice. then your best strategy would be to take the first roll if its outcome is more than its expected value (ie 3.5) and to roll again if it is less.
Expected payoff of a dice game:
Description: You have the option to throw a die up to three times. You will earn the face value of the die. You have the option to stop after each throw and walk away with the money earned. The earnings are not additive. What is the expected payoff of this game?
Rolling twice: $$\frac{1}{6}(6+5+4) + \frac{1}{2}3.5 = 4.25.$$
Rolling three times: $$\frac{1}{6}(6+5) + \frac{2}{3}4.25 = 4 + \frac{2}{3}$$
-
-
math.stackexchange.com math.stackexchange.com
-
Therefore, En=2n+1−2=2(2n−1)
Simplified formula for the expected number of tosses (e) to get
nconsecutive heads(n≥1):$$e_n=2(2^n-1)$$
For example, to get 5 consecutive heads, we've to toss the coin 62 times:
$$e_n=2(2^5-1)=62$$
We can also start with the longer analysis of the 5 scenarios:
- If we get a tail immediately (probability 1/2) then the expected number is e+1.
- If we get a head then a tail (probability 1/4), then the expected number is e+2.
- If we get two head then a tail (probability 1/8), then the expected number is e+2.
- If we get three head then a tail (probability 1/16), then the expected number is e+4.
- If we get four heads then a tail (probability 1/32), then the expected number is e+5.
- Finally, if our first 5 tosses are heads, then the expected number is 5.
Thus:
$$e=\frac{1}{2}(e+1)+\frac{1}{4}(e+2)+\frac{1}{8}(e+3)+\frac{1}{16}\\(e+4)+\frac{1}{32}(e+5)+\frac{1}{32}(5)=62$$
We can also generalise the formula to:
$$e_n=\frac{1}{2}(e_n+1)+\frac{1}{4}(e_n+2)+\frac{1}{8}(e_n+3)+\frac{1}{16}\\(e_n+4)+\cdots +\frac{1}{2^n}(e_n+n)+\frac{1}{2^n}(n) $$
-
-
blog.jessitron.com blog.jessitron.com
-
In math, idempotence describes only unary functions that you can call on their own output. Math-idempotence is, “If you take the absolute value of a number, and then you take the absolute value of that, the result doesn’t change on the second (or subsequent) operations.” Math.abs is math-idempotent. Math-idempotence only applies to functions of one parameter where the parameter type and return type are the same. Not so useful in programming.
-
Programming-idempotence is about side effects. It’s about stuff that happens to the outside world when you call a function. Idempotence says “If you’ve called me once, it doesn’t matter whether you called me again.”
-
-
tauday.com tauday.com
-
Tau Day is an annual celebration of the circle constant τ=6.283185…\tau = 6.283185\ldots, which takes place every June 28 (6/28 in the American calendar system).
Tau (τ) = 2π radians = 360°
It's simply a more intuitive way of representing a full circular rotation. Possibly Euler could've changed it 100 years ago, but too many textbooks already applied the concept of 2π radians instead
-
- Mar 2020
-
amitness.com amitness.com
-
This denotes the factorial of a number. It is the product of numbers starting from 1 to that number.
Exclamation in Python: $$x!$$ is written as:
x = 5 fact = 1 for i in range(x, 0, -1): fact = fact * i print(fact)it can be shortened as:
import math math.factorial(x)and the output is:
# 5*4*3*2*1 120 -
The hat gives the unit vector. This means dividing each component in a vector by it’s length(norm).
Hat in Python: $$\hat{x}$$ is written as:
x = [1, 2, 3] length = math.sqrt(sum([e**2 for e in x])) x_hat = [e/length for e in x]This makes the magnitude of the vector 1 and only keeps the direction:
math.sqrt(sum([e**2 for e in x_hat])) # 1.0 -
It gives the sum of the products of the corresponding entries of the two sequences of numbers.
Dot Product in Python: $$X.Y$$ is written as:
X = [1, 2, 3] Y = [4, 5, 6] dot = sum([i*j for i, j in zip(X, Y)]) # 1*4 + 2*5 + 3*6 # 32 -
It means multiplying the corresponding elements in two tensors. In Python, this would be equivalent to multiplying the corresponding elements in two lists.
Element wise multiplication in Python: $$z=x\odot y$$ is written as:
x = [[1, 2], [3, 4]] y = [[2, 2], [2, 2]] z = np.multiply(x, y)and results in:
[[2, 4]], [[6, 8]] -
This is basically exchanging the rows and columns.
Transpose in Python: $$X^T$$ is written as:
X = [[1, 2, 3], [4, 5, 6]] np.transpose(X)and results in:
[[1, 4], [2, 5], [3, 6]] -
This denotes a function which takes a domain X and maps it to range Y. In Python, it’s equivalent to taking a pool of values X, doing some operation on it to calculate pool of values Y.
Function in Python: $$f:X \rightarrow Y$$ is written as:
def f(X): Y = ... return YUsing
Rinstead ofXorYmeans we're dealing with real numbers: $$f:R \rightarrow R$$ then,R^2means we're dealing with d-dimensional vector of real numbers (in this case, example ofd=2isX = [1,2] -
This symbol checks if an element is part of a set.
Epilson in Python: $$3 \epsilon X$$ is written as:
X = {1,2,3} 3 in X -
The norm is used to calculate the magnitude of a vector. In Python, this means squaring each element of an array, summing them and then taking the square root.
Norm of vector in Python (it's like Pythagorean theorem): $$| x|$$ is written as:
x = [1, 2, 3] math.sqrt(x[0]**2 + x[1]**2 + x[2]**2) -
This symbol denotes the absolute value of a number i.e. without a sign.
Absolute value in Python: $$|x|$$ is written as:
x = 10 y = -20 abs(x) #10 abs(y) #20 -
In Python, it is equivalent to looping over a vector from index 0 to index N-1 and multiplying them.
PI in Python is the same as sigma, but you multiply (*) the numbers inside the for loop. $$\prod_{i=1}^Nx^i$$
-
we reuse the sigma notation and divide by the number of elements to get an average.
Average in Python: $$\frac{1}{N}\sum_{i=1}^Nx_i$$ is written as:
x = [1, 2, 3, 4, 5] result = 0 N = len(x) for i in range(n): result = result + x[i] average = result / N print(average)or it can be shortened:
x = [1, 2, 3, 4, 5] result = sum(x) / len(x) -
In Python, it is equivalent to looping over a vector from index 0 to index N-1
Sigma in Python: $$\sum_{i=1}^Nx_i$$ is written as:
x = [1, 2, 3, 4, 5] result = 0 N = len(x) for i in range(N): result = result + x[i] print(result)or it can be shortened:
x = [1, 2, 3, 4, 5] result = sum(x) -
2D vectors
2D vectors in Python: $$x_{ij}$$ are written as:
x = [ [10, 20, 30], [40, 50, 60] ] i = 0 j = 1 print(x[i][j]) # 20
Tags
Annotators
URL
-
-
datagenetics.com datagenetics.com
-
This volume of paper should be the same as the coaxial plug of paper on the roll.
Calculating volume of the paper roll: $$\mathbf{Lwt = \pi w(R^2 - r^2)} \~\ L = \text{length of the paper} \ w = \text{width of the paper} \ t = \text{thickness} \ R = \text{outer radius} \ r = \text{inner radius}$$ And that simplifies into a formula for R: $$\color{red} {\bf R = \sqrt{\frac{Lt}{\pi}+r^2}}$$
Tags
Annotators
URL
-
- Feb 2020
-
-
During our lives we learn that numbers are linear, that the spaces between them are all the same. If you count from one to nine, each number is one more than the previous one. If you ask someone what number is halfway between one and nine, they will say five—but only because they have been taught to. Wake up, sheeple! Humans instinctively perceive numbers logarithmically, not linearly. A young child or someone who has not been indoctrinated by education will place three halfway between one and nine.
Logarithmic middle: middle with respect to multiplication rather than addition --> why do we do this by default
-
- Jan 2020
-
www.youtube.com www.youtube.com
-
Good interview. not about AI. ideas in math
Tags
Annotators
URL
-
- Sep 2019
-
theconversation.com theconversation.com
-
P vs NP completion problems
-
- Jul 2019
-
docdrop.org docdrop.org
-
One major idea in mathematics is the idea of “closure”. This is the ques-tion: What is the set of all things that can result from my proposed oper-ations? In the case of vectors: What is the set of vectors that can result bystarting with a small set of vectors, and adding them to each other andscaling them? This results in a vector space
closure in mathematics. sounds similar to domain of a function
-
-
en.wikipedia.org en.wikipedia.org
-
In Hardy's words, "Exposition, criticism, appreciation, is work for second-rate minds. [...] It is a melancholy experience for a professional mathematician to find himself writing about mathematics. The function of a mathematician is to do something, to prove new theorems, to add to mathematics, and not to talk about what he or other mathematicians have done."
similar to Nassim Taleb's "History is written by losers"
Tags
Annotators
URL
-
- May 2019
-
www.businessinsider.com www.businessinsider.com
-
If you do the math
Here is the math: 2020-2023 4 years (Jan 1-Dec 31). 1 million annual is 4 million. That is way more than the 1.7 million
-
four
3 million vehicles divided by 4 years divided by 500,000 per factory makes less than two factories in my book.
-
- Mar 2019
-
sprott.physics.wisc.edu sprott.physics.wisc.edu
-
pi = 3.1415 ... e = 2.718 ... Euler's constant, gamma = 0.577215 ... = lim n -> infinity > (1 + 1/2 + 1/3 + 1/4 + ... + 1/n - ln(n)) (Not proven to be transcendental, but generally believed to be by mathematicians.) Catalan's constant, G = sum (-1)^k / (2k + 1 )^2 = 1 - 1/9 + 1/25 - 1/49 + ... (Not proven to be transcendental, but generally believed to be by mathematicians.) Liouville's number 0.110001000000000000000001000 ... which has a one in the 1st, 2nd, 6th, 24th, etc. places and zeros elsewhere. Chaitin's "constant", the probability that a random algorithm halts. (Noam Elkies of Harvard notes that not only is this number transcendental but it is also incomputable.) Chapernowne's number, 0.12345678910111213141516171819202122232425... This is constructed by concatenating the digits of the positive integers. (Can you see the pattern?) Special values of the zeta function, such as zeta (3). (Transcendental functions can usually be expected to give transcendental results at rational points.) ln(2). Hilbert's number, 2(sqrt 2 ). (This is called Hilbert's number because the proof of whether or not it is transcendental was one of Hilbert's famous problems. In fact, according to the Gelfond-Schneider theorem, any number of the form ab is transcendental where a and b are algebraic (a ne 0, a ne 1 ) and b is not a rational number. Many trigonometric or hyperbolic functions of non-zero algebraic numbers are transcendental.) epi pie (Not proven to be transcendental, but generally believed to be by mathematicians.) Morse-Thue's number, 0.01101001 ... ii = 0.207879576... (Here i is the imaginary number sqrt(-1). Isn't this a real beauty? How many people have actually considered rasing i to the i power? If a is algebraic and b is algebraic but irrational then ab is transcendental. Since i is algebraic but irrational, the theorem applies. Note also: ii is equal to e(- pi / 2 ) and several other values. Consider ii = e(i log i ) = e( i times i pi / 2 ) . Since log is multivalued, there are other possible values for ii. Here is how you can compute the value of ii = 0.207879576... 1. Since e^(ix) = Cos x + i Sin x, then let x = Pi/2. 2. Then e^(iPi/2) = i = Cos Pi/2 + i Sin Pi/2; since Cos Pi/2 = Cos 90 deg. = 0. But Sin 90 = 1 and i Sin 90 deg. = (i)*(1) = i. 3. Therefore e^(iPi/2) = i. 4. Take the ith power of both sides, the right side being i^i and the left side = [e^(iPi/2)]^i = e^(-Pi/2). 5. Therefore i^i = e^(-Pi/2) = .207879576... Feigenbaum numbers, e.g. 4.669 ... . (These are related to properties of dynamical systems with period-doubling. The ratio of successive differences between period-doubling bifurcation parameters approaches the number 4.669 ... , and it has been discovered in many physical systems before they enter the chaotic regime. It has not been proven to be transcendental, but is generally believed to be.)
Tags
Annotators
URL
-
- Jan 2019
-
en.wikipedia.org en.wikipedia.org
-
A rooted binary tree is full if every vertex has either two children or no children.
Example of Catalan Numbers use case.
Tags
Annotators
URL
-
-
en.wikipedia.org en.wikipedia.org
-
Catalan numbers notation and short explanation of it.
Use - LookUP: Combinatorics (non crossing combinations) ex: ((())), ()(()), ()()(), (())(), (()())
ref: https://www.geeksforgeeks.org/program-nth-catalan-number/
-
- Dec 2018
-
www.npr.org www.npr.org
-
computer owned by Patrick Laroche of Ocala, Fla., discovered the number on Dec. 7,
Newest Mersenne Prime was discovered in Ocala, FL, very close to us.
Interesting tidbit :)
-
- Nov 2018
-
www.coursera.org www.coursera.org
-
Basic Statistics
part of specialization
Tags
Annotators
URL
-
-
www.coursera.org www.coursera.org
-
Mathematics for Machine Learning
specialization
-
-
meduza.io meduza.io
-
Начните с нескольких основных книг, которые изменят ваши представления о математикеЛучше всего «ставит голову» книга «Начала теории множеств» Николая Верещагина и Александра Шеня. Она даст основу для понимания (а в дальнейшем и самостоятельного построения!) логических рассуждений. С нее же начнется и понимание теории множеств, лежащей в основе современной математики.Охватить больше разделов математики поможет книга Рихарда Куранта и Герберта Роббинса «Что такое математика?». Как и книгу Верещагина и Шеня, эту книгу нужно читать внимательно, делая все упражнения. Если первые две книги окажутся сложными, можете начать с моей «Математики для гуманитариев». Ее также следует читать с самого начала, страницу за страницей, не стоит браться за чтение с середины. Она не очень простая, но предварительных сведений и математической культуры не предполагает.Чтобы понять, каким образом математика входит в нашу жизнь, можно прочитать «Кому нужна математика?» Андрея Райгородского и Нелли Литвак или «Математическую составляющую» (сборник сюжетов под редакцией Николая Андреева).
nice books
-
- Sep 2018
-
blog.emacsen.net blog.emacsen.net
-
Learning Math Earlier With Computers
-
- Apr 2018
-
www.tandfonline.com www.tandfonline.com
-
we found advanced math and science courses advertised but not actually offered and specialized STEM programs eroded or discontinued within a few years of inception.
Some interesting data points in this article.
-
-
www.edweek.org www.edweek.org
-
Yet, across the country, 2 in 5 high schools don't offer physics, according to an Education Week Research Center analysis of data from the U.S. Department of Education's office for civil rights.
How widely known is this figure?
-
-
-
n this note we determine thetorsion subgroup of the additive group of (1.1) in the case whereLhas rank 2, thatisXis a set of two elements, andcis a prime number
Wow!
Tags
Annotators
URL
-
-
link.springer.com link.springer.com
-
Of particular interest are the lower central series: G1=GG1=GG_1 =G, Gi+1=[Gi,G]Gi+1=[Gi,G]G_{i+1} = [G_i, G], i≥1i≥1i \ge 1, and, for a fixed prime number p, the Zassenhaus series (see [7, 8]).
This is interesting.
-
-
www.exploratorium.edu www.exploratorium.edu
-
Leonhard Euler, who adopted it in 1737
Actually, he first used π in 1727, and it meant 6.28.., not 3.14..
Tags
Annotators
URL
-
